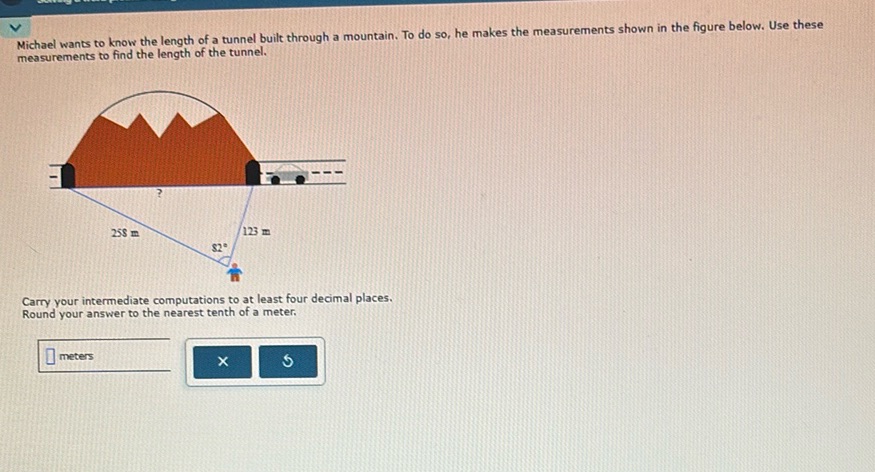
Question: Michael wants to know the length of a tunnel built through a mountain. To do so, he makes the…

Michael wants to know the length of a tunnel built through a mountain. To do so, he makes the measurements shown in the figure below. Use these measurements to find the length of the tunnel.

[Diagram of a mountain with a tunnel through it. The tunnel is shown as a straight line passing through the mountain. There are two lines extending from the tunnel entrance to a point on the ground, forming a triangle. The lengths of these lines are 258 m and 123 m, and the angle between them is 82 degrees.]

Carry your intermediate computations to at least four decimal places. Round your answer to the nearest tenth of a meter.

[Input field for meters]

[Buttons for submit and clear]

Solution

To solve the problem of finding the length of the tunnel, we can use the Law of Cosines. The problem provides us the lengths of two sides of a triangle (258 m and 123 m) and the angle between them (52°). Let’s denote: - \( a = 258 \) m (one side of the triangle) - \( b = 123 \) m (the other side of the triangle) - \( C = 52^\circ \) (the angle opposite the tunnel) - \( c \) is the length of the tunnel we need to find. The Law of Cosines is given by: \[ c^2 = a^2 + b^2 - 2ab \cdot \cos(C) \] Substitute the known values into the equation: \[ c^2 = 258^2 + 123^2 - 2 \cdot 258 \cdot 123 \cdot \cos(52^\circ) \] Calculate \( 258^2 \): \[ 258^2 = 66564 \] Calculate \( 123^2 \): \[ 123^2 = 15129 \] Calculate the cosine term: \[ 2 \cdot 258 \cdot 123 \cdot \cos(52^\circ) \approx 2 \cdot 258 \cdot 123 \cdot 0.6157 \approx 19665.9462 \] Substitute these values back into the equation: \[ c^2 = 66564 + 15129 - 19665.9462 \] Simplify the equation: \[ c^2 = 62027.0538 \] Take the square root to find \( c \): \[ c \approx \sqrt{62027.0538} \approx 248.0503 \] Rounding \( c \) to the nearest tenth of a meter: The length of the tunnel is approximately \( 248.1 \) meters.