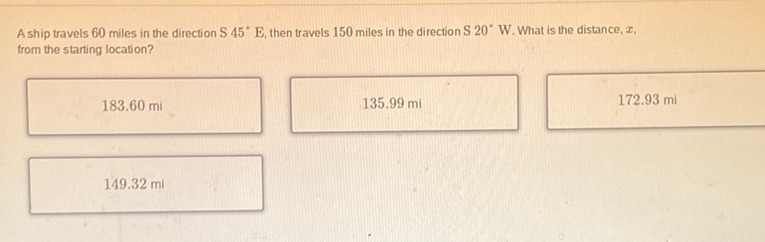
Question: A ship travels 60 miles in the direction S 45° E, then travels 150 miles in the direction S 20° W…

A ship travels 60 miles in the direction S 45° E, then travels 150 miles in the direction S 20° W. What is the distance, \( z \), from the starting location?

183.60 mi 135.99 mi 172.93 mi 149.32 mi

Solution

To solve the problem, we will use vector addition and trigonometry to determine the ship’s final displacement from its starting point. First, let’s define a coordinate system where the positive \( x \)-axis points east and the positive \( y \)-axis points north. The ship makes two movements: 1. Travels 60 miles in the direction S 45° E. 2. Travels 150 miles in the direction S 20° W. Step 1: Resolve the first movement into its \( x \) and \( y \) components. \[ \text{First movement:} \] \[ x_1 = 60 \times \sin(45^\circ) & \] \[ y_1 = -60 \times \cos(45^\circ) & \] Step 2: Resolve the second movement into its \( x \) and \( y \) components. \[ \text{Second movement:} \] \[ x_2 = -150 \times \sin(20^\circ) & \] \[ y_2 = -150 \times \cos(20^\circ) & \] Step 3: Calculate the total \( x \) and \( y \) components by adding the respective components of both movements. \[ \text{Total displacement:} \] \[ x_{\text{total}} = x_1 + x_2 & \] \[ y_{\text{total}} = y_1 + y_2 & \] Step 4: Compute the numerical values using the sine and cosine of the given angles. \[ x_{\text{total}} = 60 \times 0.7071 - 150 \times 0.3420 \approx -8.874 \text{ miles} & \] \[ y_{\text{total}} = -60 \times 0.7071 - 150 \times 0.9397 \approx -183.381 \text{ miles} & \] Step 5: Determine the distance \( x \) from the starting location using the Pythagorean theorem. \[ x = \sqrt{(x_{\text{total}})^2 + (y_{\text{total}})^2} & \] \[ x = \sqrt{(-8.874)^2 + (-183.381)^2} \approx 183.60 \text{ miles} & \] Answer: 183.60 mi