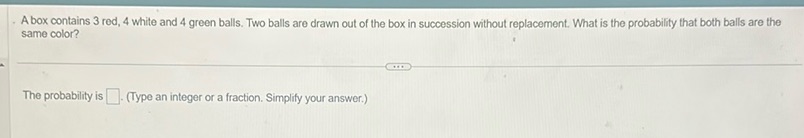
Question: A box contains 3 red, 4 white and 4 green balls. Two balls are drawn out of the box in succession…

A box contains 3 red, 4 white and 4 green balls. Two balls are drawn out of the box in succession without replacement. What is the probability that both balls are the same color? The probability is (Type an integer or a fraction. Simplify your answer.)

Solution

To find the probability that both balls drawn are the same color, follow these steps: There are 3 red, 4 white, and 4 green balls, making a total of 11 balls. First, calculate the probability of drawing two red balls: The probability of the first ball being red is \[ \frac{3}{11} \] The probability of the second ball being red, after one red is already drawn (without replacement), is \[ \frac{2}{10} = \frac{1}{5} \] The probability of both being red is \[ \frac{3}{11} \times \frac{1}{5} = \frac{3}{55} \] Next, calculate the probability of drawing two white balls: The probability of the first ball being white is \[ \frac{4}{11} \] The probability of the second ball being white, after one white is already drawn, is \[ \frac{3}{10} \] The probability of both being white is \[ \frac{4}{11} \times \frac{3}{10} = \frac{12}{110} = \frac{6}{55} \] Then, calculate the probability of drawing two green balls: The probability of the first ball being green is \[ \frac{4}{11} \] The probability of the second ball being green, after one green is already drawn, is \[ \frac{3}{10} \] The probability of both being green is \[ \frac{4}{11} \times \frac{3}{10} = \frac{12}{110} = \frac{6}{55} \] Finally, add the probabilities of both balls being the same color: \[ \frac{3}{55} + \frac{6}{55} + \frac{6}{55} = \frac{15}{55} = \frac{3}{11} \] The probability that both balls are the same color is \[ \frac{3}{11} \]