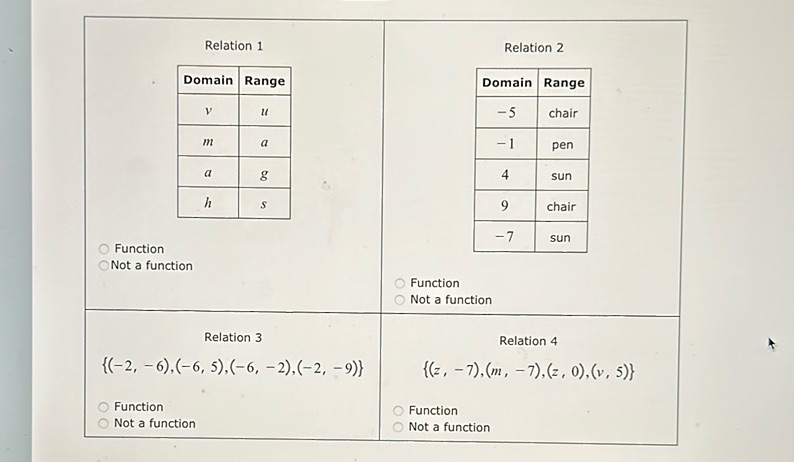
Question: ### Transcription #### Relation 1 - Domain | Range - \( v \) | \( u \) - \( m \)…

Transcription

Relation 1

• Domain | Range

  • \( v \) | \( u \)
  • \( m \) | \( a \)
  • \( a \) | \( g \)
  • \( h \) | \( s \)

• Function

• Not a function

Relation 2

• Domain | Range

  • \( -5 \) | chair
  • \( -1 \) | pen
  • \( 4 \) | sun
  • \( 9 \) | chair
  • \( -7 \) | sun

• Function

• Not a function

Relation 3

• \(\{(-2, -6), (-6, 5), (-6, -2), (-2, -9)\}\)

• Function

• Not a function

Relation 4

• \(\{(z, -7), (m, -7), (z, 0), (v, 5)\}\)

• Function

• Not a function

Solution

To determine whether each relation is a function, check if each element from the domain is related to exactly one element from the range. Relation 1: - Domain and Range: - \( v \rightarrow u \) - \( m \rightarrow a \) - \( a \rightarrow g \) - \( h \rightarrow s \) Each element of the domain is mapped to one element in the range. Relation 1 is a function. Relation 2: - Domain and Range: - \(-5 \rightarrow \text{chair} \) - \(-1 \rightarrow \text{pen} \) - \( 4 \rightarrow \text{sun} \) - \( 9 \rightarrow \text{chair} \) - \(-7 \rightarrow \text{sun} \) Each element of the domain is mapped to one element in the range. Relation 2 is a function. Relation 3: - Set of pairs: - \((-2, -6), (-6, 5), (-6, -2), (-2, -9) \) The element \(-2\) is associated with both \(-6\) and \(-9\), and \(-6\) is associated with both \(5\) and \(-2\). Relation 3 is not a function. Relation 4: - Set of pairs: - \((z, -7), (m, -7), (z, 0), (v, 5) \) The element \(z\) is associated with both \(-7\) and \(0\). Relation 4 is not a function.