Published
- 1 min read
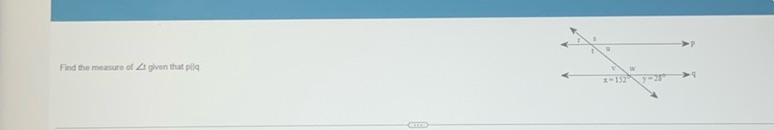
Question: Find the measure of \( \angle A \) given that \( p \parallel q \). \[ \begin{array}{c}…
Find the measure of \( \angle A \) given that \( p \parallel q \).
\[ \begin{array}{c} \angle A = 122^\circ \\ \angle B = 28^\circ \\ \angle C = 30^\circ \end{array} \]
Solution
Find the measure of \(\angle 1\) given that \(p \parallel q\). From the diagram, \(\angle 1\) and \((2x - 25)^\circ\) are corresponding angles. Since \(p \parallel q\), corresponding angles are equal. \[ \angle 1 = (2x - 25)^\circ \] Also, from the diagram, we know: \[ x + 152^\circ = 180^\circ \] Subtract \(152\) degrees from both sides to find \(x\): \[ x = 180^\circ - 152^\circ \] \[ x = 28^\circ \] Now substitute \(x = 28^\circ\) back into the equation for \(\angle 1\): \[ \angle 1 = 2(28) - 25 \] \[ \angle 1 = 56 - 25 \] \[ \angle 1 = 31^\circ \] Therefore, the measure of \(\angle 1\) is \(31^\circ\).

