Question: Exercise 9 1. For the hyperbola given by $9x^2 - 16y^2 = 144$, find the standard form of the…

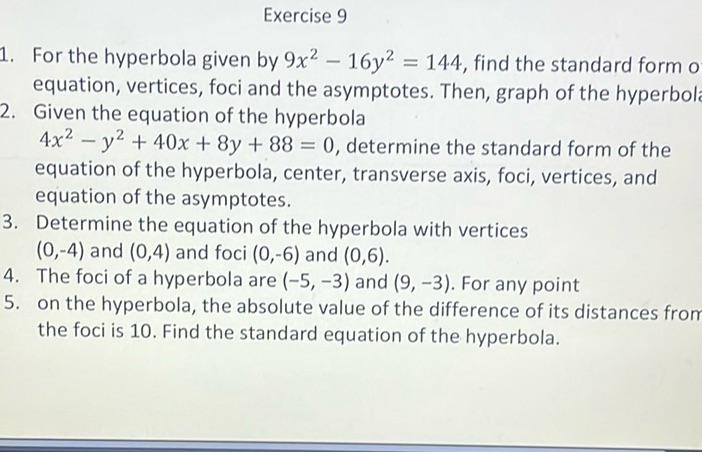
Exercise 9

1. For the hyperbola given by $9x^2 - 16y^2 = 144$, find the standard form of the equation, vertices, foci, and the asymptotes. Then, graph the hyperbola.

2. Given the equation of the hyperbola $4x^2 - y^2 + 40x + 8y + 88 = 0$, determine the standard form of the equation of the hyperbola, center, transverse axis, foci, vertices, and equation of the asymptotes.

3. Determine the equation of the hyperbola with vertices $(0, -4)$ and $(0, 4)$ and foci $(0, -6)$ and $(0, 6)$.

4. The foci of a hyperbola are $(-5, -3)$ and $(9, -3)$. For any point on the hyperbola, the absolute value of the difference of its distances from the foci is 10. Find the standard equation of the hyperbola.

Solution

For problem 1, the hyperbola is given by: \[ 9x^2 - 16y^2 = 144 \] First, divide the entire equation by 144 to normalize it. \[ \frac{9x^2}{144} - \frac{16y^2}{144} = 1 \] Simplify the fractions: \[ \frac{x^2}{16} - \frac{y^2}{9} = 1 \] This is in the standard form \(\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1\), where \(a^2 = 16\) and \(b^2 = 9\). Now find the vertices. The vertices are located at \((\pm a, 0)\). Since \(a^2 = 16\), \(a = 4\). Vertices: \((4, 0)\) and \((-4, 0)\). Find the foci. The formula for the foci of a hyperbola is \(c^2 = a^2 + b^2\). Calculate \(c^2\): \[ c^2 = 16 + 9 = 25 \] So, \(c = 5\). Foci: \((5, 0)\) and \((-5, 0)\). Determine the asymptotes. The equations of the asymptotes are \(y = \pm \frac{b}{a}x\). Thus, \[ y = \pm \frac{3}{4}x \] This completes the information on the standard form, vertices, foci, and asymptotes of the hyperbola.