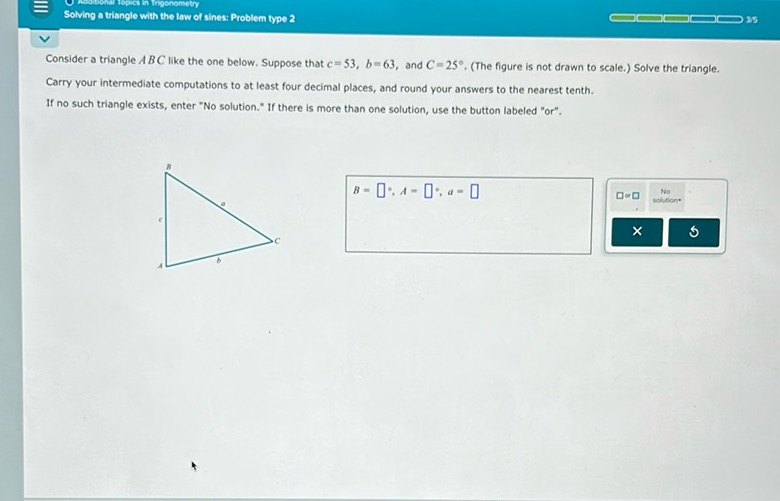
Question: Consider a triangle \( \triangle ABC \) like the one below. Suppose that \( c = 53 \), \( b =…

Consider a triangle \( \triangle ABC \) like the one below. Suppose that \( c = 53 \), \( b = 63 \), and \( C = 25^\circ \). (The figure is not drawn to scale.) Solve the triangle.

Carry your intermediate computations to at least four decimal places, and round your answers to the nearest tenth.

If no such triangle exists, enter “No solution.” If there is more than one solution, use the button labeled “or”.

[Diagram of triangle \( \triangle ABC \) with sides \( a \), \( b \), and \( c \) and angles \( A \), \( B \), and \( C \)]

\( B = \boxed{\phantom{0}}^\circ \), \( A = \boxed{\phantom{0}}^\circ \), \( a = \boxed{\phantom{0}} \)

[Buttons for “No solution” and “or”]

Solution

To solve the triangle using the given information, we will apply the law of sines: Given: - \( c = 53 \) - \( b = 63 \) - \( C = 25^\circ \) Use the law of sines: \[ \frac{b}{\sin B} = \frac{c}{\sin C} \] Substitute the known values to find \( \sin B \): \[ \frac{63}{\sin B} = \frac{53}{\sin 25^\circ} \] \[ \sin B = \frac{63 \cdot \sin 25^\circ}{53} \] Calculate \(\sin 25^\circ\) and then find \(\sin B\): \(\sin 25^\circ \approx 0.4226\) \[ \sin B = \frac{63 \cdot 0.4226}{53} \] \[ \sin B \approx 0.5016 \] Find angle \( B \): \[ B = \sin^{-1}(0.5016) \approx 30.1^\circ \] Now use the angle sum of a triangle to find \( A \): \[ A + B + C = 180^\circ \] \[ A + 30.1^\circ + 25^\circ = 180^\circ \] \[ A = 180^\circ - 30.1^\circ - 25^\circ \] \[ A = 124.9^\circ \] Finally, use the law of sines to find side \( a \): \[ \frac{a}{\sin A} = \frac{c}{\sin C} \] \[ \frac{a}{\sin 124.9^\circ} = \frac{53}{\sin 25^\circ} \] \[ \sin 124.9^\circ \approx 0.8387 \] \[ a = \frac{53 \cdot 0.8387}{0.4226} \] \[ a \approx 105.1 \] So, the solutions are: \[ B \approx 30.1^\circ, \quad A \approx 124.9^\circ, \quad a \approx 105.1 \]