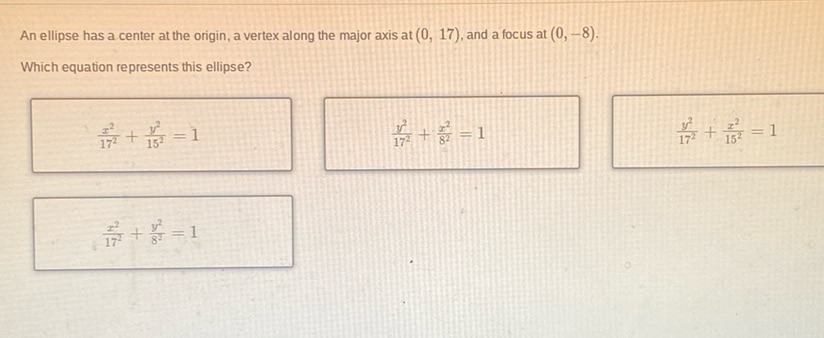
Question: An ellipse has a center at the origin, a vertex along the major axis at (0, 17), and a focus at (0,…

An ellipse has a center at the origin, a vertex along the major axis at (0, 17), and a focus at (0, -8).

Which equation represents this ellipse?

1. \(\frac{x^2}{17^2} + \frac{y^2}{15^2} = 1\)

2. \(\frac{y^2}{17^2} + \frac{x^2}{8^2} = 1\)

3. \(\frac{y^2}{17^2} + \frac{x^2}{15^2} = 1\)

4. \(\frac{x^2}{17^2} + \frac{y^2}{8^2} = 1\)

Solution

The problem states that an ellipse has a center at the origin \((0, 0)\), a vertex along the major axis at \((0, 17)\), and a focus at \((0, -8)\). This indicates a vertically oriented ellipse. The general form of a vertical ellipse centered at the origin is: \[ \frac{x^2}{b^2} + \frac{y^2}{a^2} = 1 \] where \(a > b\), and the foci are at \((0, \pm c)\), with \(c^2 = a^2 - b^2\). Step 1: Identify \(a\) Since the vertex is at \((0, 17)\), we have \(a = 17\). Step 2: Identify \(c\) The focus is at \((0, -8)\), so \(c = 8\). Step 3: Use the relationship \(c^2 = a^2 - b^2\) to find \(b^2\). \[ c^2 = a^2 - b^2 \] \[ 8^2 = 17^2 - b^2 \] \[ 64 = 289 - b^2 \] Step 4: Solve for \(b^2\) \[ b^2 = 289 - 64 \] \[ b^2 = 225 \] Therefore, the equation of the ellipse is: \[ \frac{x^2}{225} + \frac{y^2}{289} = 1 \] So the correct answer is: \[ \frac{y^2}{17^2} + \frac{x^2}{15^2} = 1 \]