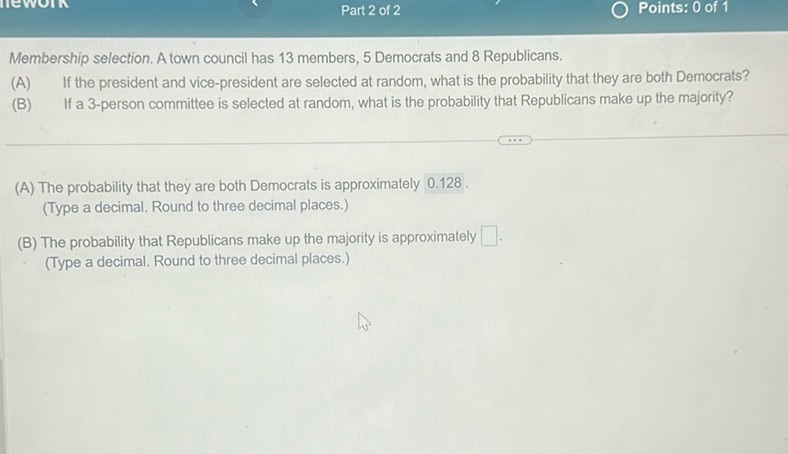
Question: Membership selection. A town council has 13 members, 5 Democrats and 8 Republicans. (A) If the…

Membership selection. A town council has 13 members, 5 Democrats and 8 Republicans. (A) If the president and vice-president are selected at random, what is the probability that they are both Democrats? (B) If a 3-person committee is selected at random, what is the probability that Republicans make up the majority?

(A) The probability that they are both Democrats is approximately 0.128. (Type a decimal. Round to three decimal places.) (B) The probability that Republicans make up the majority is approximately . (Type a decimal. Round to three decimal places.)

Solution

To solve part (B) of the problem, we need to calculate the probability that Republicans make up the majority in a 3-person committee. This means at least two out of the three members selected should be Republicans. First, let’s find the combinations where Republicans form the majority: 1. Two Republicans and one Democrat: - Number of ways to choose 2 Republicans out of 8: \[ \binom{8}{2} = \frac{8 \times 7}{2 \times 1} = 28 \] - Number of ways to choose 1 Democrat out of 5: \[ \binom{5}{1} = 5 \] - Total ways to choose 2 Republicans and 1 Democrat: \[ 28 \times 5 = 140 \] 2. Three Republicans: - Number of ways to choose 3 Republicans out of 8: \[ \binom{8}{3} = \frac{8 \times 7 \times 6}{3 \times 2 \times 1} = 56 \] Now, compute the total ways to select any 3-person committee from 13 members: \[ \binom{13}{3} = \frac{13 \times 12 \times 11}{3 \times 2 \times 1} = 286 \] Next, calculate the probability that Republicans are the majority: - Total favorable outcomes: \[ 140 + 56 = 196 \] - Probability that Republicans are the majority: \[ \frac{196}{286} \approx 0.685 \] Therefore, the probability that Republicans make up the majority is approximately 0.685.