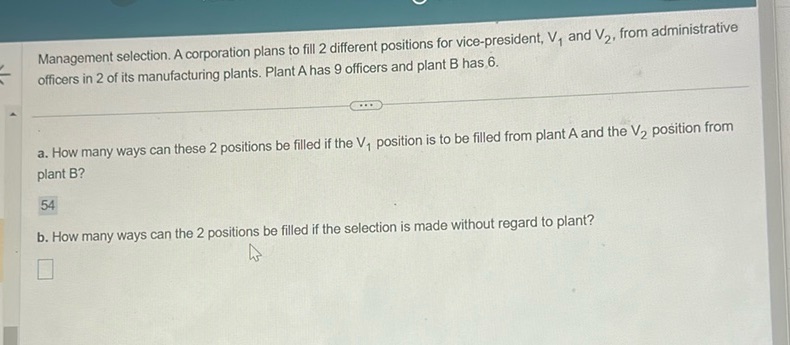
Question: Management selection. A corporation plans to fill 2 different positions for vice-president, V₁ and…

Management selection. A corporation plans to fill 2 different positions for vice-president, V₁ and V₂, from administrative officers in 2 of its manufacturing plants. Plant A has 9 officers and plant B has 6.

a. How many ways can these 2 positions be filled if the V₁ position is to be filled from plant A and the V₂ position from plant B? 54 b. How many ways can the 2 positions be filled if the selection is made without regard to plant?

Solution

a. To find the number of ways to fill the two positions \( V_1 \) and \( V_2 \) where \( V_1 \) is from plant A and \( V_2 \) is from plant B: - Plant A has 9 officers to choose from for position \( V_1 \). - Plant B has 6 officers to choose from for position \( V_2 \). The total number of combinations is: \[ 9 \times 6 = 54 \] b. To find the number of ways to fill the two positions without regard to which plant the officers come from: - There are a total of 15 officers (9 from plant A and 6 from plant B). - We need to choose 2 officers for the 2 different positions. The number of ways to choose 2 officers out of 15 is calculated using permutations, since the positions are different: \[ 15 \times 14 = 210 \] Therefore, there are 210 ways to fill the positions without regard to plant.