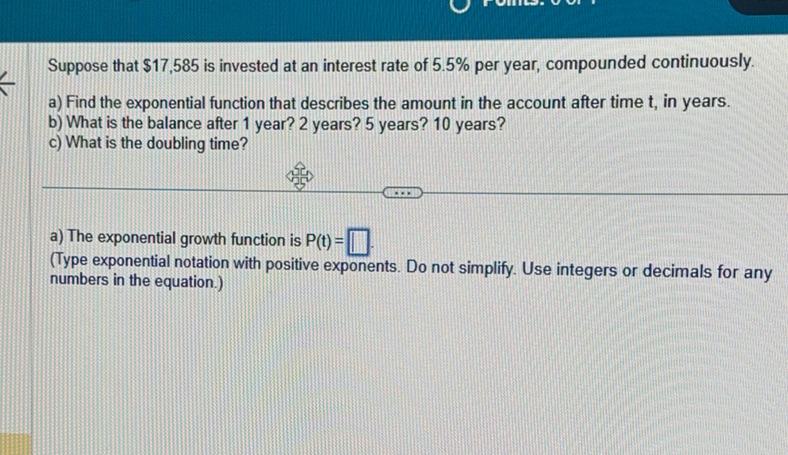
Question: Suppose that $17,585 is invested at an interest rate of 5.5% per year, compounded continuously. a)…

Suppose that $17,585 is invested at an interest rate of 5.5% per year, compounded continuously.

a) Find the exponential function that describes the amount in the account after time t, in years. b) What is the balance after 1 year? 2 years? 5 years? 10 years? c) What is the doubling time?

a) The exponential growth function is P(t) = [\boxed{\quad}]. (Type exponential notation with positive exponents. Do not simplify. Use integers or decimals for any numbers in the equation.)

Solution

To solve the problem, we’ll use the formula for continuous compounding interest: \[ P(t) = P_0 \cdot e^{rt} \] where: - \(P(t)\) is the amount after time \(t\), - \(P_0\) is the initial amount (principal), - \(r\) is the interest rate, - \(e\) is the base of the natural logarithm, - \(t\) is the time in years. Given: - \(P_0 = 17585\) - \(r = 0.055\) ### a) Exponential Function Substitute the given values into the formula: \[ P(t) = 17585 \cdot e^{0.055t} \] ### b) Balance After 1, 2, 5, 10 Years Calculate \(P(t)\) for the given values of \(t\): For \(t = 1\): \[ P(1) = 17585 \cdot e^{0.055 \cdot 1} = 17585 \cdot e^{0.055} \] For \(t = 2\): \[ P(2) = 17585 \cdot e^{0.055 \cdot 2} = 17585 \cdot e^{0.11} \] For \(t = 5\): \[ P(5) = 17585 \cdot e^{0.055 \cdot 5} = 17585 \cdot e^{0.275} \] For \(t = 10\): \[ P(10) = 17585 \cdot e^{0.055 \cdot 10} = 17585 \cdot e^{0.55} \] ### c) Doubling Time The doubling time \(T\) can be found using the formula: \[ T = \frac{\ln(2)}{r} \] Substitute \(r = 0.055\): \[ T = \frac{\ln(2)}{0.055} \] This will give the time required for the investment to double in value.