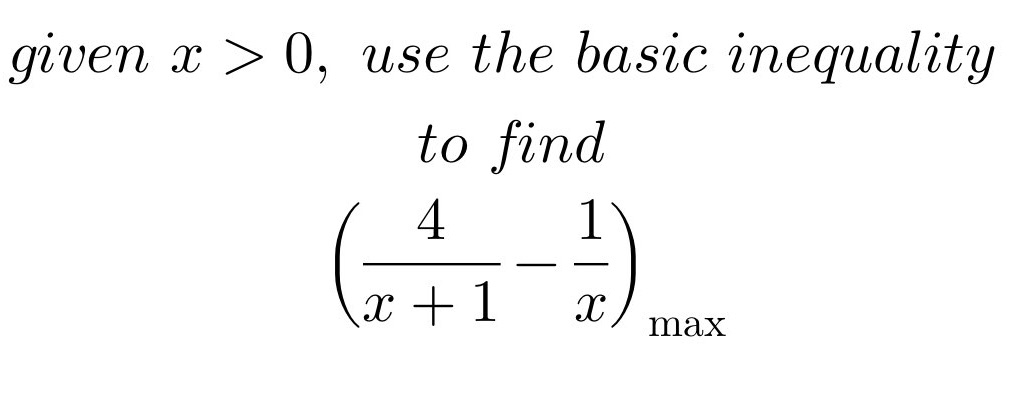
Question: Given \( x > 0 \), use the basic inequality to find \[ \left( \frac{4}{x + 1} - \frac{1}{x}…

Given \( x > 0 \), use the basic inequality to find \[ \left( \frac{4}{x + 1} - \frac{1}{x} \right)_{\text{max}} \]

Solution

To solve this problem, we can use the basic inequality known as the AM-GM inequality. The Arithmetic Mean - Geometric Mean Inequality states that for any non-negative real numbers \(a\) and \(b\), \[ \frac{a + b}{2} \geq \sqrt{ab} \] We want to maximize: \[ f(x) = \frac{4}{x+1} - \frac{1}{x} \] First, find a common denominator: \[ f(x) = \frac{4x - (x+1)}{x(x+1)} = \frac{3x - 1}{x(x+1)} \] Now, consider the expression \(3x - 1\). We apply the first derivative test to find critical points. Calculate the derivative \(f’(x)\): \[ f(x) = \frac{3x - 1}{x(x+1)} \] Using the quotient rule, \(\left(\frac{u}{v}\right)’ = \frac{u’v - uv’}{v^2}\), where \( u = 3x - 1 \) and \( v = x(x+1) \). Derivatives are: \( u’ = 3 \) \( v’ = x + 1 + x = 2x + 1 \) \[ f’(x) = \frac{3(x^2 + x) - (3x - 1)(2x + 1)}{(x(x+1))^2} \] Simplify the derivative: \[ f’(x) = \frac{3x^2 + 3x - (6x^2 + 3x - 2x - 1)}{x^2(x + 1)^2} \] \[ f’(x) = \frac{3x^2 + 3x - 6x^2 - x + 1}{x^2(x + 1)^2} \] \[ f’(x) = \frac{-3x^2 + 2x + 1}{x^2(x + 1)^2} \] Set this to zero to find critical points: \[ -3x^2 + 2x + 1 = 0 \] Solve using the quadratic formula, \(x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\), where \(a = -3\), \(b = 2\), \(c = 1\). \[ x = \frac{-2 \pm \sqrt{2^2 - 4(-3)(1)}}{2(-3)} \] \[ x = \frac{-2 \pm \sqrt{4 + 12}}{-6} \] \[ x = \frac{-2 \pm \sqrt{16}}{-6} \] \[ x = \frac{-2 \pm 4}{-6} \] Solutions are: \[ x = \frac{2}{6} = \frac{1}{3} \quad \text{and} \quad x = \frac{-6}{-6} = 1 \] Check the function at critical points and endpoints (as \(x \to 0^+\) and \(x \to \infty\)). Evaluate \(f(x)\) at these points: - As \(x \to 0^+\), \(f(x) \to -\infty\). - At \(x = \frac{1}{3}\), \[ f\left(\frac{1}{3}\right) = \frac{3(\frac{1}{3}) - 1}{(\frac{1}{3})(\frac{1}{3} + 1)} = \frac{1 - 1}{\frac{1}{3}(\frac{4}{3})} = 0 \] - At \(x = 1\), \[ f(1) = \frac{3(1) - 1}{1(1+1)} = \frac{2}{2} = 1 \] - As \(x \to \infty\), \(f(x) \to 0\). The maximum value of the function is at \(x = 1\). Therefore, the maximum value of \(\frac{4}{x+1} - \frac{1}{x}\) is \(1\).