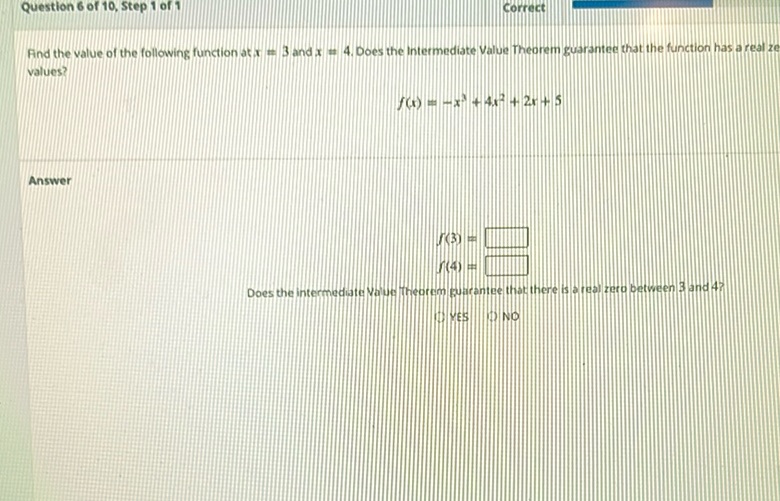
Question: Find the value of the following function at \( x = 3 \) and \( x = 4 \). Does the Intermediate…

Find the value of the following function at \( x = 3 \) and \( x = 4 \). Does the Intermediate Value Theorem guarantee that the function has a real zero between these values?

\[ f(x) = -x^3 + 4x^2 + 2x + 5 \]

Answer:

\[ f(3) = \boxed{\phantom{0}} \] \[ f(4) = \boxed{\phantom{0}} \]

Does the Intermediate Value Theorem guarantee that there is a real zero between 3 and 4?

• YES
• NO

Solution

To solve the given problem, we’ll follow the steps below: Calculate \( f(3) \): \[ f(3) = -3^3 + 4 \times 3^2 + 2 \times 3 + 5 & \] \[ f(3) = -27 + 36 + 6 + 5 & \] \[ f(3) = 20 & \] Calculate \( f(4) \): \[ f(4) = -4^3 + 4 \times 4^2 + 2 \times 4 + 5 & \] \[ f(4) = -64 + 64 + 8 + 5 & \] \[ f(4) = 13 & \] Now, analyze whether the Intermediate Value Theorem guarantees a real zero between 3 and 4: Since \( f(3) = 20 \) and \( f(4) = 13 \), both \( f(3) \) and \( f(4) \) are positive. The Intermediate Value Theorem requires that the function takes on both positive and negative values in the interval to guarantee a real zero. Answer: NO