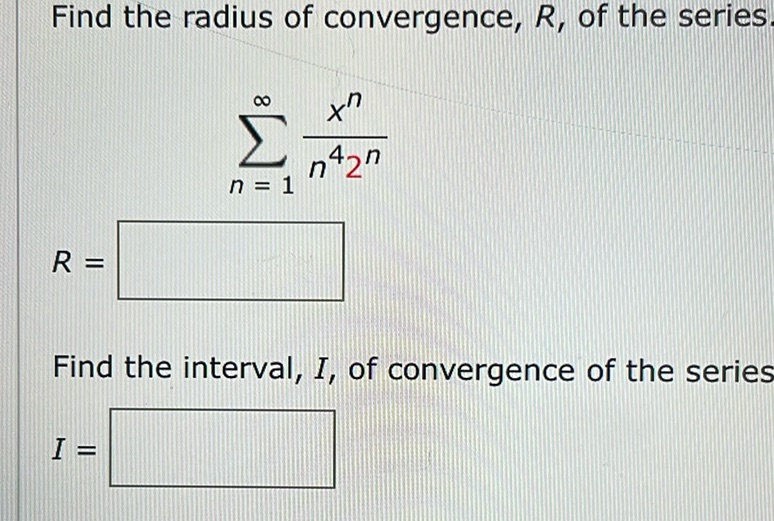
Question: Find the radius of convergence, \( R \), of the series. \[ \sum_{n=1}^{\infty}…

Find the radius of convergence, \( R \), of the series.

\[ \sum_{n=1}^{\infty} \frac{x^n}{n^4 2^n} \]

\( R = \)

Find the interval, \( I \), of convergence of the series.

\( I = \)

Solution

To find the radius of convergence \( R \) and the interval of convergence \( I \) for the series: \[ \sum_{n=1}^{\infty} \frac{x^n}{n^4 2^n} \] Finding the Radius of Convergence \( R \): We can use the Root Test for determining the radius of convergence. \[ \lim_{n \to \infty} \sqrt[n]{\left| \frac{x^n}{n^4 2^n} \right|} = \lim_{n \to \infty} \frac{|x|^{1}}{2} = \frac{|x|}{2} \] For convergence, this limit must be less than 1: \[ \frac{|x|}{2} < 1 \] \[ |x| < 2 \] Thus, the radius of convergence is: \[ R = 2 \] Finding the Interval of Convergence \( I \): Given that \( R = 2 \), the interval is: \[ -2 < x < 2 \] We need to check the endpoints \( x = -2 \) and \( x = 2 \). 1. At \( x = 2 \): \[ \sum_{n=1}^{\infty} \frac{2^n}{n^4 2^n} = \sum_{n=1}^{\infty} \frac{1}{n^4} \] This is a p-series with \( p = 4 > 1 \), which converges. 2. At \( x = -2 \): \[ \sum_{n=1}^{\infty} \frac{(-2)^n}{n^4 2^n} = \sum_{n=1}^{\infty} \frac{(-1)^n}{n^4} \] This is an alternating p-series with \( p = 4 > 1 \), which converges. Therefore, the interval of convergence is: \[ I = [-2, 2] \]