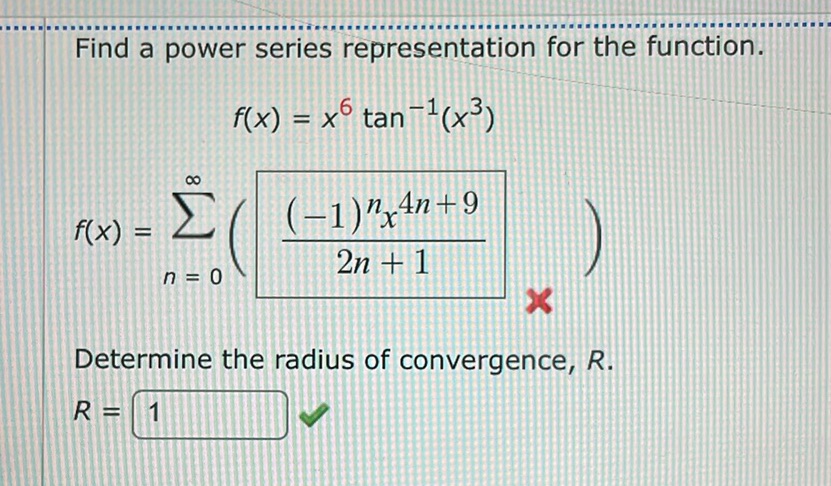
Question: Find a power series representation for the function. \[ f(x) = x^6 \tan^{-1}(x^3) \] \[ f(x)…

Find a power series representation for the function.

\[ f(x) = x^6 \tan^{-1}(x^3) \]

\[ f(x) = \sum_{n=0}^{\infty} \left( \frac{(-1)^n x^{4n+9}}{2n+1} \right) \]

Determine the radius of convergence, \( R \).

\[ R = 1 \]

Solution

To solve the problem of finding the power series representation for the function \( f(x) = x^6 \tan^{-1}(x^3) \) and determining its radius of convergence, follow the steps below: Step 1: Start with the given function. \[ f(x) = x^6 \tan^{-1}(x^3) \] Step 2: Recall the power series expansion for \( \tan^{-1}(x) \): \[ \tan^{-1}(x) = \sum_{n=0}^{\infty} \frac{(-1)^n x^{2n+1}}{2n+1} \quad \text{for} \ |x| \leq 1 \] Step 3: Substitute \( x^3 \) into the power series of \( \tan^{-1}(x) \): \[ \tan^{-1}(x^3) = \sum_{n=0}^{\infty} \frac{(-1)^n (x^3)^{2n+1}}{2n+1} = \sum_{n=0}^{\infty} \frac{(-1)^n x^{6n+3}}{2n+1} \] Step 4: Multiply the series by \( x^6 \) to obtain the power series for \( f(x) \): \[ f(x) = x^6 \tan^{-1}(x^3) = x^6 \sum_{n=0}^{\infty} \frac{(-1)^n x^{6n+3}}{2n+1} = \sum_{n=0}^{\infty} \frac{(-1)^n x^{6n+9}}{2n+1} \] Step 5: Determine the radius of convergence \( R \). The original power series for \( \tan^{-1}(x) \) converges for \( |x| \leq 1 \). Since we substituted \( x^3 \) into the series, the condition becomes: \[ |x^3| \leq 1 \implies |x| \leq 1 \] Thus, the radius of convergence is: \[ R = 1 \]