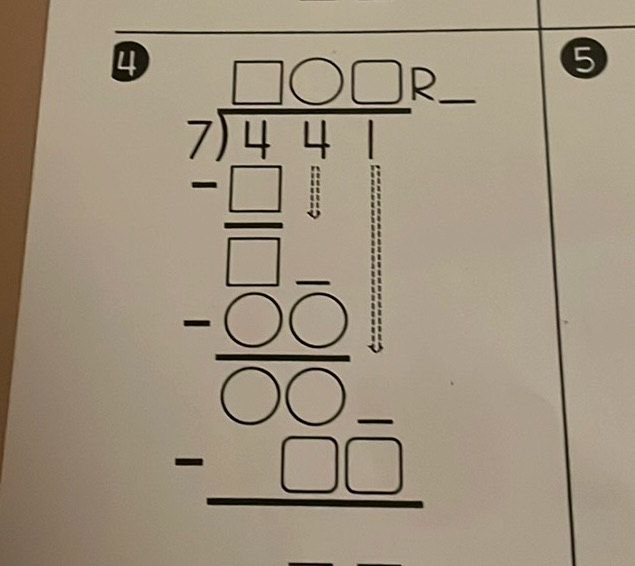
Question: 4. \(\frac{7}{441}\) 5. \(\frac{7}{441}\)

Solution

To solve this division problem: Let’s perform long division of \(441\) by \(7\). First, divide \(44\) by \(7\). - \(7\) goes into \(44\) six times (since \(7 \times 6 = 42\)). \[ \begin{aligned} &\phantom{44} - 42 \\ \end{aligned} \] Subtract \(42\) from \(44\) to get \(2\). Bring down the next digit \(1\) to make \(21\). - \(7\) goes into \(21\) three times (since \(7 \times 3 = 21\)). \[ \begin{aligned} &\phantom{21} - 21 \\ \end{aligned} \] Subtract \(21\) from \(21\) to get \(0\). So, \(441\) divided by \(7\) is \(63\) with a remainder of \(0\). Final Answer: The quotient is \(63\), and the remainder is \(0\).