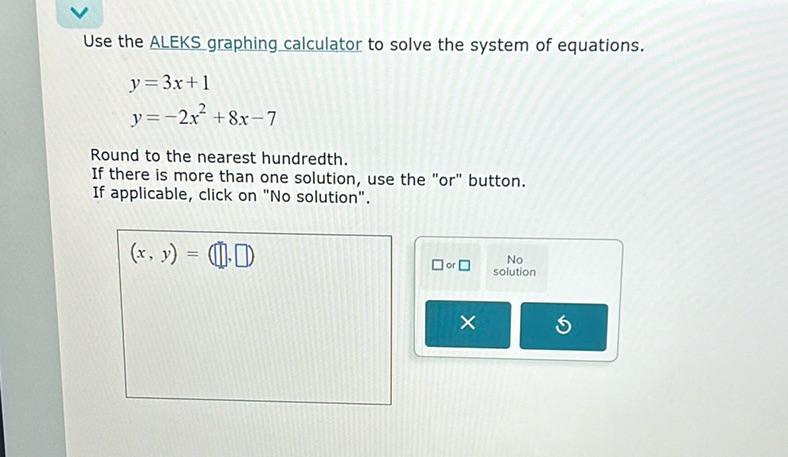
Question: Use the ALEKS graphing calculator to solve the system of equations. \[ y = 3x + 1 \] \[ y =…

Use the ALEKS graphing calculator to solve the system of equations.

\[ y = 3x + 1 \] \[ y = -2x^2 + 8x - 7 \]

Round to the nearest hundredth.

If there is more than one solution, use the “or” button.

If applicable, click on “No solution”.

\((x, y) = (\boxed{\phantom{00}}, \boxed{\phantom{00}})\)

Solution

To solve the system of equations using algebra, substitute the expression for \( y \) from the first equation into the second equation. Given: \[ \begin{cases} y = 3x + 1 & \\ y = -2x^2 + 8x - 7 & \end{cases} \] Substitute \( y = 3x + 1 \) into \( y = -2x^2 + 8x - 7 \): \[ 3x + 1 = -2x^2 + 8x - 7 \] Rearrange the equation: \[ 2x^2 + 3x - 8x + 1 + 7 = 0 \] Simplify: \[ 2x^2 - 5x + 8 = 0 \] Now, find the roots of the quadratic equation using the quadratic formula: The quadratic formula is \(\frac{{-b \pm \sqrt{{b^2 - 4ac}}}}{{2a}}\). For \(2x^2 - 5x + 8 = 0\): \(a = 2\), \(b = -5\), \(c = 8\). First, calculate the discriminant: \[ b^2 - 4ac = (-5)^2 - 4 \cdot 2 \cdot 8 = 25 - 64 = -39 \] Since the discriminant is negative, the quadratic equation has no real solutions. Therefore, the system of equations has no solution.