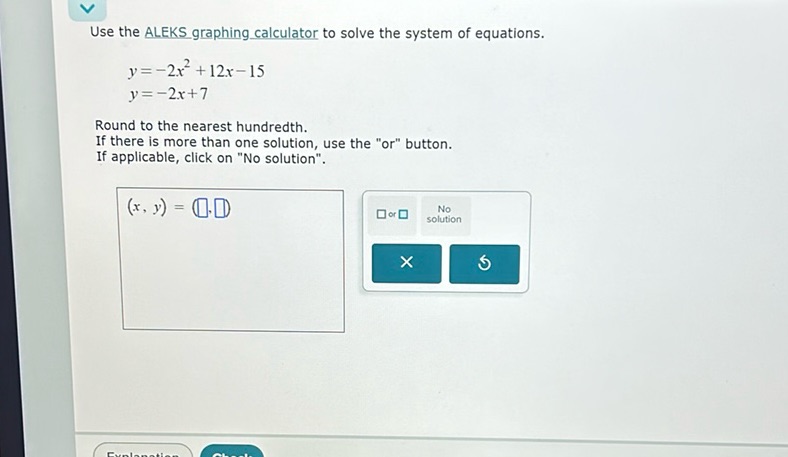
Question: Use the ALEKS graphing calculator to solve the system of equations. \[ y = -2x^2 + 12x - 15 \]…

Use the ALEKS graphing calculator to solve the system of equations.

\[ y = -2x^2 + 12x - 15 \] \[ y = -2x + 7 \]

Round to the nearest hundredth.

If there is more than one solution, use the “or” button.

If applicable, click on “No solution”.

\[ (x, y) = (\boxed{\phantom{0}}, \boxed{\phantom{0}}) \]

Solution

To solve the system of equations: \[ \begin{cases} y = -2x^2 + 12x - 15 & \\ y = -2x + 7 & \end{cases} \] Set the equations equal to each other: \[ -2x^2 + 12x - 15 = -2x + 7 \] Rearrange all terms to one side: \[ -2x^2 + 12x - 15 + 2x - 7 = 0 \] Simplify the equation: \[ -2x^2 + 14x - 22 = 0 \] Divide the entire equation by -2 to simplify: \[ x^2 - 7x + 11 = 0 \] Use the quadratic formula \(x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\) where \(a = 1\), \(b = -7\), and \(c = 11\): Calculate the discriminant \(b^2 - 4ac\): \[ (-7)^2 - 4(1)(11) = 49 - 44 = 5 \] Calculate the roots: \[ x = \frac{-(-7) \pm \sqrt{5}}{2(1)} = \frac{7 \pm \sqrt{5}}{2} \] So, the solutions for \(x\) are: \[ x_1 = \frac{7 + \sqrt{5}}{2} \quad \text{and} \quad x_2 = \frac{7 - \sqrt{5}}{2} \] Calculate the corresponding \(y\) values using \(y = -2x + 7\). For \(x_1 = \frac{7 + \sqrt{5}}{2}\): \[ y_1 = -2\left(\frac{7 + \sqrt{5}}{2}\right) + 7 = -7 - \sqrt{5} + 7 = -\sqrt{5} \] For \(x_2 = \frac{7 - \sqrt{5}}{2}\): \[ y_2 = -2\left(\frac{7 - \sqrt{5}}{2}\right) + 7 = -7 + \sqrt{5} + 7 = \sqrt{5} \] Rounded to the nearest hundredth: \[ x_1 \approx 5.62, \quad y_1 \approx -2.24 \] \[ x_2 \approx 1.38, \quad y_2 \approx 2.24 \] Thus, the solutions are: \[ (x, y) = (5.62, -2.24) \quad \text{or} \quad (1.38, 2.24) \]