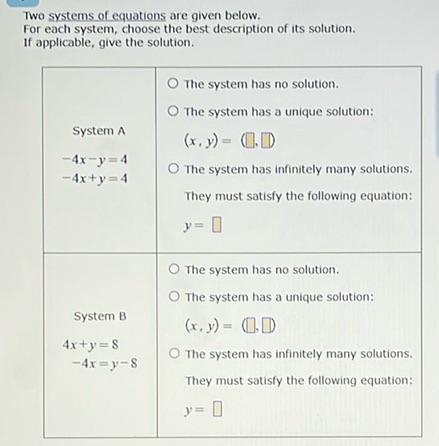
Question: Two systems of equations are given below. For each system, choose the best description of its…

Two systems of equations are given below. For each system, choose the best description of its solution. If applicable, give the solution.

System A \[ \begin{align*} -4x - y &= 4 \\ -4x + y &= 4 \end{align*} \]

• The system has no solution.
• The system has a unique solution: \((x, y) = (\boxed{\phantom{0}}, \boxed{\phantom{0}})\)
• The system has infinitely many solutions. They must satisfy the following equation: \(y = \boxed{\phantom{0}}\)

System B \[ \begin{align*} 4x + y &= 8 \\ -4x &= y - 8 \end{align*} \]

• The system has no solution.
• The system has a unique solution: \((x, y) = (\boxed{\phantom{0}}, \boxed{\phantom{0}})\)
• The system has infinitely many solutions. They must satisfy the following equation: \(y = \boxed{\phantom{0}}\)

Solution

For System A: The system of equations is: \[ \begin{cases} -4x - y = 4 & \\ -4x + y = 4 & \end{cases} \] Add the two equations to eliminate \( y \): \[ (-4x - y) + (-4x + y) = 4 + 4 \] \[ -8x = 8 \] Divide by -8: \[ x = -1 \] Substitute \( x = -1 \) back into the first equation: \[ -4(-1) - y = 4 \] \[ 4 - y = 4 \] Subtract 4 from both sides: \[ -y = 0 \] Multiply by -1: \[ y = 0 \] The system has a unique solution: \((x, y) = (-1, 0)\). --- For System B: The system of equations is: \[ \begin{cases} 4x + y = 8 & \\ -4x = y - 8 & \end{cases} \] Substitute \( y = 4x + 8 \) from the second equation into the first: \[ 4x + (4x + 8) = 8 \] \[ 8x + 8 = 8 \] Subtract 8 from both sides: \[ 8x = 0 \] Divide by 8: \[ x = 0 \] Substitute \( x = 0 \) back into the equation for \( y \): \[ y = 4(0) + 8 \] \[ y = 8 \] The system has a unique solution: \((x, y) = (0, 8)\).