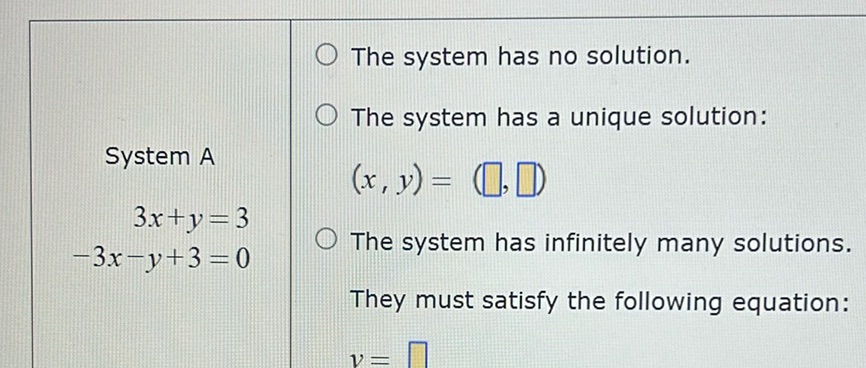
Question: The system has no solution. The system has a unique solution: \[ (x, y) = (\boxed{\phantom{0}},…

The system has no solution.

The system has a unique solution: \[ (x, y) = (\boxed{\phantom{0}}, \boxed{\phantom{0}}) \]

The system has infinitely many solutions. They must satisfy the following equation: \[ y = \boxed{\phantom{0}} \]

System A \[ 3x + y = 3 \\ -3x - y + 3 = 0 \]

Solution

To solve the given system of equations: \[ \begin{cases} 3x + y = 3 & \\ -3x - y + 3 = 0 & \end{cases} \] Add the two equations to eliminate \(x\) and \(y\). \[ (3x + y) + (-3x - y + 3) = 3 + 0 \] Simplify the left side: \[ 3x + y - 3x - y + 3 = 3 \] Which simplifies to: \[ 3 = 3 \] This is a true statement, not an equation for \(x\) or \(y\). Subtract the first equation from the second to see if there are infinitely many solutions or none: \[ (-3x - y + 3) - (3x + y) = 0 - 3 \] Simplify the left side: \[ -3x - y + 3 - 3x - y = -3 \] Combine like terms: \[ -6x - 2y + 3 = -3 \] Subtract 3 from both sides: \[ -6x - 2y = -6 \] Divide the entire equation by \(-2\): \[ 3x + y = 3 \] This is the same as the first equation, confirming they are dependent. Therefore, the system has infinitely many solutions satisfying: \[ y = -3x + 3 \]