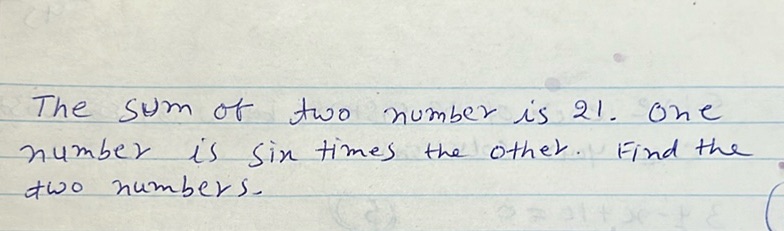
Question: The sum of two numbers is 21. One number is six times the other. Find the two numbers.

The sum of two numbers is 21. One number is six times the other. Find the two numbers.

Solution

To solve the problem, let’s denote the two numbers as \( x \) and \( y \). According to the problem, the sum of the two numbers is 21: \[ x + y = 21 \] One number is six times the other. Let’s assume \( x = 6y \). Now we have the system of equations: \[ \begin{cases} x + y = 21 & \\ x = 6y & \end{cases} \] Substitute \( x = 6y \) into the first equation: \[ 6y + y = 21 \] Combine the terms: \[ 7y = 21 \] Solve for \( y \): \[ y = \frac{21}{7} \] \[ y = 3 \] Now substitute \( y = 3 \) back into the equation \( x = 6y \): \[ x = 6 \times 3 \] \[ x = 18 \] The two numbers are 18 and 3.