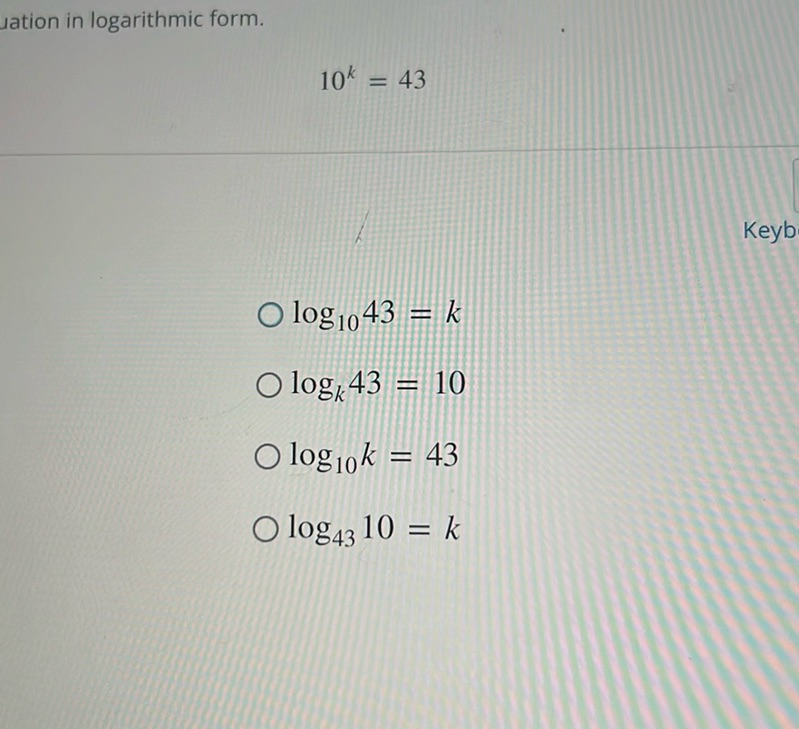
Question: The image contains a mathematical problem and its options. The problem is to convert the equation…

The image contains a mathematical problem and its options. The problem is to convert the equation \(10^k = 43\) into logarithmic form. The options are:

1. \(\log_{10} 43 = k\)
2. \(\log_k 43 = 10\)
3. \(\log_{10} k = 43\)
4. \(\log_{43} 10 = k\)

Solution

To convert the equation \(10^k = 43\) into logarithmic form, we use the definition of a logarithm: \[ \log_{\text{base}}(\text{result}) = \text{exponent} \] Here, the base is 10, the result is 43, and the exponent is \(k\). So, the logarithmic form is: \[ \log_{10}43 = k \] Therefore, the correct option is \( \log_{10}43 = k \).