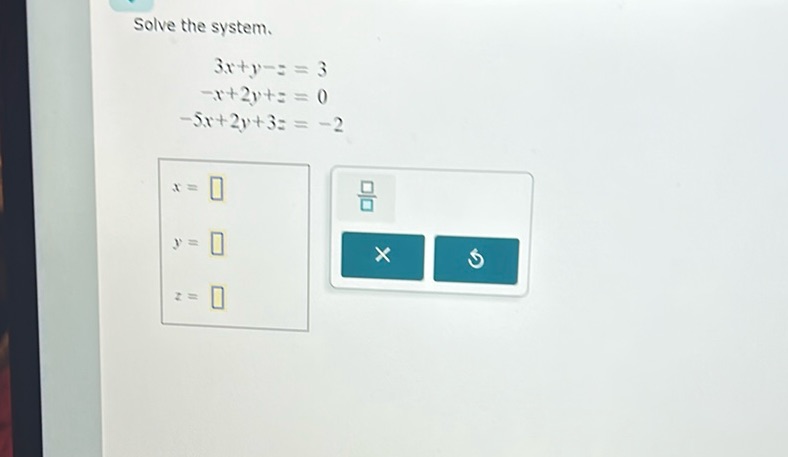
Question: Solve the system. \[ \begin{align*} 3x + y - z &= 3 \\ -x + 2y + z &= 0 \\ -5x + 2y + 3z &=…

Solve the system.

\[ \begin{align*} 3x + y - z &= 3 \\ -x + 2y + z &= 0 \\ -5x + 2y + 3z &= -2 \end{align*} \]

\( x = \boxed{\phantom{0}} \)

\( y = \boxed{\phantom{0}} \)

\( z = \boxed{\phantom{0}} \)

Solution

To solve the system of equations: \[ \begin{cases} 3x + y - z = 3 & \\ -x + 2y + z = 0 & \\ -5x + 2y + 3z = -2 & \end{cases} \] First, let’s eliminate \(z\) by adding the first and second equations. Add equations 1 and 2: \( (3x + y - z) + (-x + 2y + z) = 3 + 0 \) \[ 2x + 3y = 3 \] Next, let’s eliminate \(z\) from the second and third equations. Add equations 2 and 3: \( (-x + 2y + z) + (-5x + 2y + 3z) = 0 + (-2) \) \[ -6x + 4y + 4z = -2 \] Now, simplify: \[ -3x + 2y + 2z = -1 \] Let’s now solve the system composed of the equations: \[ \begin{cases} 2x + 3y = 3 & \\ -3x + 2y + 2z = -1 & \end{cases} \] From equation 1: \[ y = \frac{3 - 2x}{3} \] Substitute \(y = \frac{3 - 2x}{3}\) into equation 3: \(-3x + 2\left(\frac{3 - 2x}{3}\right) + 2z = -1\) Solve for \(x\) and \(z\): \[ -3x + \frac{6 - 4x}{3} + 2z = -1 \] Multiply by 3 to clear denominators: \[ -9x + 6 - 4x + 6z = -3 \] Combine like terms: \[ -13x + 6z = -9 \] \[ 6z = 13x - 9 \] \[ z = \frac{13x - 9}{6} \] Substitute the expression for \(z\) back into the first set of equations if needed and solve for specific values of \(x\), \(y\), and \(z\). In this case, solving these equations will yield the solutions for \(x\), \(y\), and \(z\).