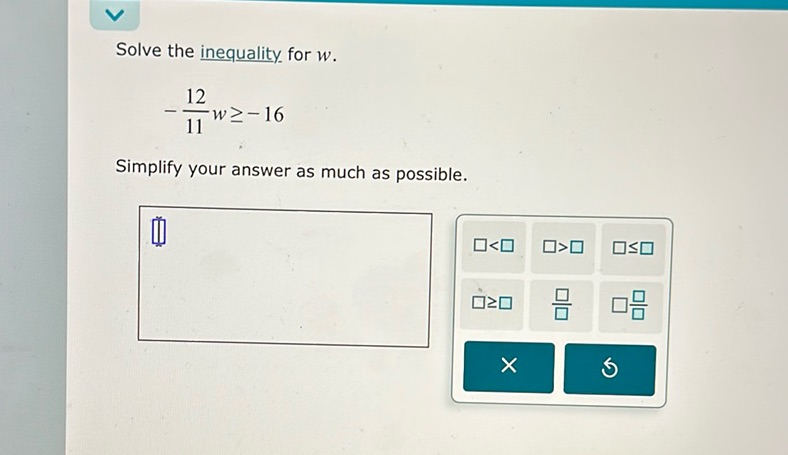
Question: Solve the inequality for w. - \frac{12}{11} w \geq -16 Simplify your answer as much as possible.

Solve the inequality for w.

• \frac{12}{11} w \geq -16 Simplify your answer as much as possible.

Solution

Solve the inequality for \( w \): \[ -\frac{12}{11} w \geq -16 \] First, multiply both sides of the inequality by \(-\frac{11}{12}\) to isolate \( w \). Remember that multiplying by a negative number reverses the inequality sign: \[ w \leq -16 \times -\frac{11}{12} \] Calculate the right side: \[ w \leq \frac{176}{12} \] Simplify the fraction: \[ w \leq \frac{44}{3} \] Thus, the solution is: \[ w \leq \frac{44}{3} \]