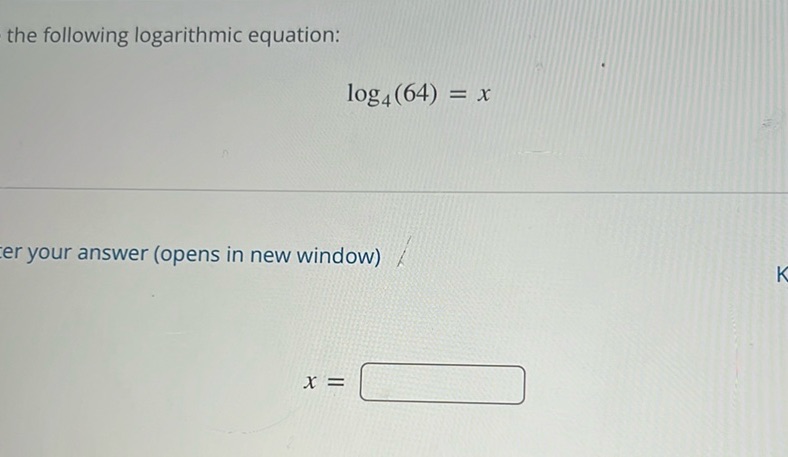
Question: Solve the following logarithmic equation: \[ \log_{4}(64) = x \] Enter your answer (opens in…

Solve the following logarithmic equation:

\[ \log_{4}(64) = x \]

Enter your answer (opens in new window)

\[ x = \boxed{\phantom{0}} \]

Solution

To solve the logarithmic equation: \[ \log_4(64) = x \] First, rewrite the logarithmic equation in exponential form. This means: \[ 4^x = 64 \] Next, express 64 as a power of 4. Since \(64 = 4^3\), we have: \[ 4^x = 4^3 \] Since the bases are the same, we equate the exponents: \[ x = 3 \] Therefore, the solution is: \[ x = 3 \]