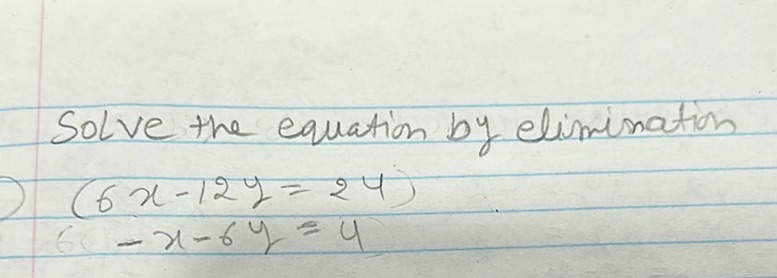
Question: Solve the equation by elimination \[ \begin{align*} (6x - 12y) &= 24 \\ 6x - 6y &= 4…

Solve the equation by elimination

\[ \begin{align*} (6x - 12y) &= 24 \\ 6x - 6y &= 4 \end{align*} \]

Solution

To solve the system of equations by elimination, follow these steps: Given: \[ \begin{cases} 6x - 12y = 24 & \\ -x - 6y = 4 & \end{cases} \] First, multiply the second equation by 6 to align the coefficients of \( x \) for elimination: \[ \begin{cases} 6x - 12y = 24 & \\ -6x - 36y = 24 & \end{cases} \] Add both equations to eliminate \( x \): \[ (6x - 12y) + (-6x - 36y) = 24 + 24 \] Simplify: \[ -48y = 48 \] Divide both sides by -48: \[ y = -1 \] Substitute \( y = -1 \) into the first original equation to solve for \( x \): \[ 6x - 12(-1) = 24 \] Simplify: \[ 6x + 12 = 24 \] Subtract 12 from both sides: \[ 6x = 12 \] Divide by 6: \[ x = 2 \] The solution to the system of equations is \( x = 2 \) and \( y = -1 \).