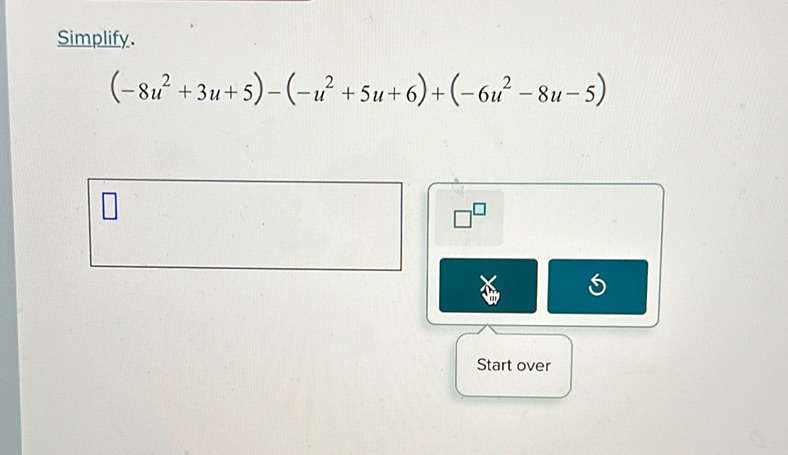
Question: Simplify. (-8u^2 + 3u + 5) - (-u^2 + 5u + 6) + (-6u^2 - 8u - 5)

Simplify. (-8u^2 + 3u + 5) - (-u^2 + 5u + 6) + (-6u^2 - 8u - 5)

Solution

To simplify the expression \[ (-8u^2 + 3u + 5) - (-u^2 + 5u + 6) + (-6u^2 - 8u - 5) \] First, distribute the negative sign in the second term: \[ = -8u^2 + 3u + 5 + u^2 - 5u - 6 + (-6u^2 - 8u - 5) \] Simplify by combining like terms: Combine the \(u^2\) terms: \[ -8u^2 + u^2 - 6u^2 = -13u^2 \] Combine the \(u\) terms: \[ 3u - 5u - 8u = -10u \] Combine the constant terms: \[ 5 - 6 - 5 = -6 \] Combine all simplified terms: \[ -13u^2 - 10u - 6 \] The simplified expression is \[ -13u^2 - 10u - 6 \]