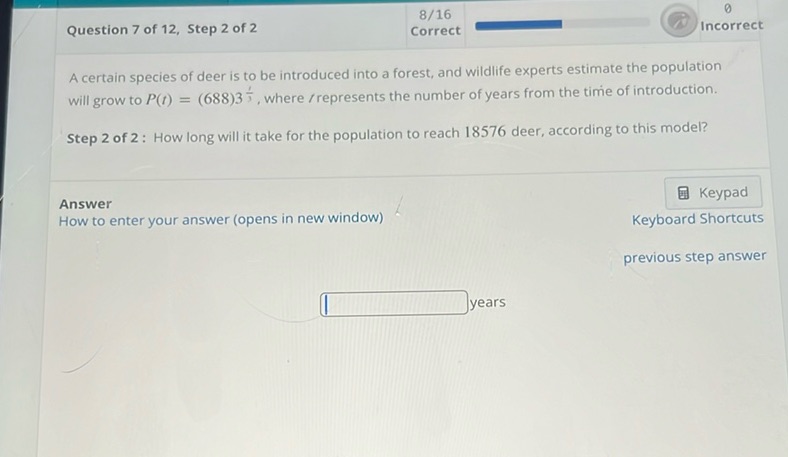
Question: Question 7 of 12, Step 2 of 2 A certain species of deer is to be introduced into a forest, and…

Question 7 of 12, Step 2 of 2

A certain species of deer is to be introduced into a forest, and wildlife experts estimate the population will grow to \( P(t) = (688)^{\frac{t}{5}} \), where \( t \) represents the number of years from the time of introduction.

Step 2 of 2: How long will it take for the population to reach 18576 deer, according to this model?

Answer

How to enter your answer (opens in new window)

Keypad

Keyboard Shortcuts

previous step answer

years

Solution

To solve the problem, we need to find the time \( t \) it takes for the deer population to reach 18,576 based on the given model. The population model is: \[ P(t) = 688 \cdot 3^{\frac{t}{5}} \] We need to set \( P(t) = 18,576 \) and solve for \( t \). \[ 688 \cdot 3^{\frac{t}{5}} = 18,576 \] Divide both sides by 688: \[ 3^{\frac{t}{5}} = \frac{18,576}{688} \] Calculate the right side: \[ 3^{\frac{t}{5}} = 27 \] Recognize that \( 27 = 3^3 \): \[ 3^{\frac{t}{5}} = 3^3 \] Since the bases are the same, we can equate the exponents: \[ \frac{t}{5} = 3 \] Multiply both sides by 5: \[ t = 15 \] Therefore, it will take 15 years for the population to reach 18,576 deer.