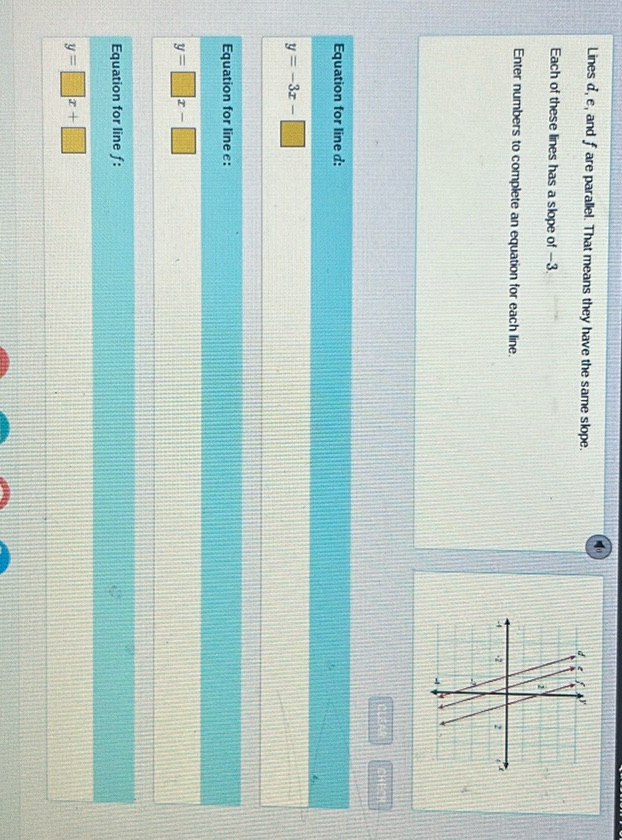
Question: Lines d, e, and f are parallel. That means they have the same slope. Each of these lines has a…

Lines d, e, and f are parallel. That means they have the same slope. Each of these lines has a slope of -3. Enter numbers to complete an equation for each line. Equation for line d: y = -3x + Equation for line e: y = -3x + Equation for line f: y = -3x +

Solution

Lines \( f, c, \) and \( d \) are parallel, which means they have the same slope of \(-3\). For each line, we need to complete the equation in the form \( y = -3x + b \). Equation for line \( f \): The equation given is \( y = -3x + \). Equation for line \( c \): The equation given is \( y = -3x - \). Equation for line \( d \): The equation given is \( y = -3x - \). Since the lines are parallel, the \( b \) values are different constants representing the y-intercepts, which you should determine based on context or further information if available.