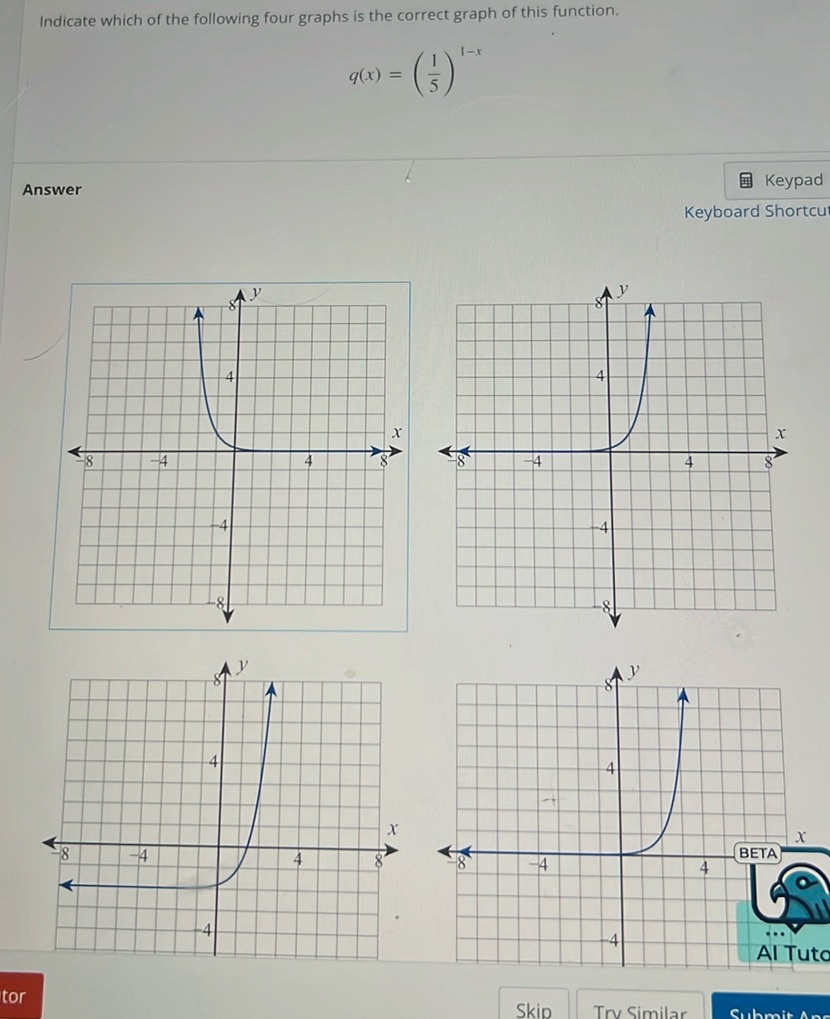
Question: Indicate which of the following four graphs is the correct graph of this function. \[ q(x) =…

Indicate which of the following four graphs is the correct graph of this function.

\[ q(x) = \left(\frac{1}{5}\right)^{1-x} \]

Answer

[Graphs are shown but not transcribed as they are visual representations.]

Solution

To determine which graph corresponds to the function \[ q(x) = \left( \frac{1}{5} \right)^{1-x} \] we follow these steps: Rewrite the function: \[ q(x) = \left( \frac{1}{5} \right)^{1-x} = \left( \frac{1}{5} \right) \cdot \left( \frac{1}{5} \right)^{-x} \] Using properties of exponents, this can be rewritten as: \[ q(x) = \frac{1}{5} \cdot 5^x \] This is an exponential function of the form \( y = c \cdot a^x \) with \( c = \frac{1}{5} \) and \( a = 5 \). Characteristics of the graph include: - As \( x \) increases, \( q(x) \) increases, since \( 5^x \) grows rapidly. - As \( x \) decreases, \( q(x) \) approaches zero but never touches the x-axis. - The graph passes through the point \( (0, \frac{1}{5}) \). Looking at the graphs, choose the one where: - The curve steeply rises as \( x \) increases. - The curve approaches zero as \( x \) decreases but never touches the x-axis. - The curve passes through approximately \( y = \frac{1}{5} \) when \( x = 0 \). The top right graph matches these characteristics.