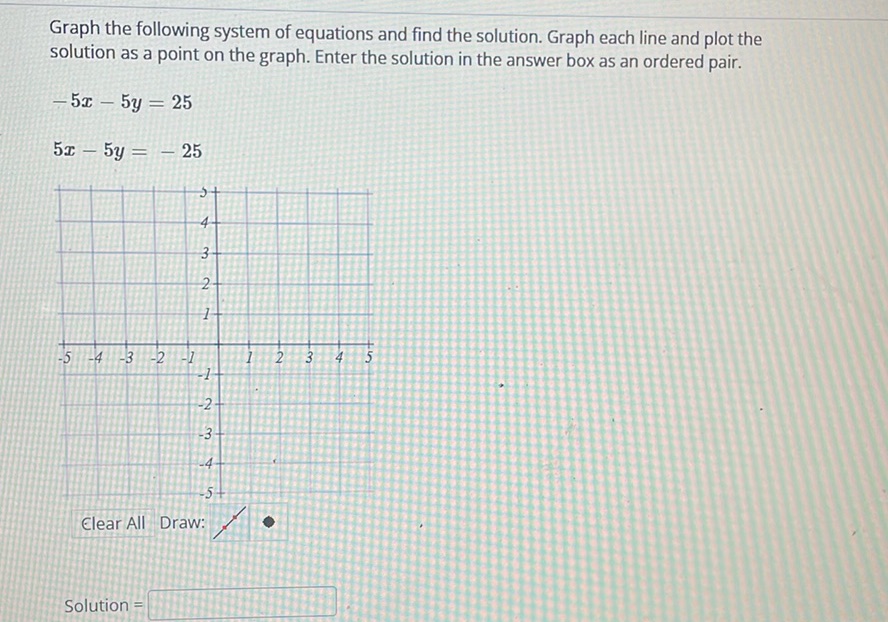
Question: Graph the following system of equations and find the solution. Graph each line and plot the…

Graph the following system of equations and find the solution. Graph each line and plot the solution as a point on the graph. Enter the solution in the answer box as an ordered pair.

\[ -5x - 5y = 25 \]

\[ 5x - 5y = -25 \]

[Graph grid]

Clear All Draw:

Solution =

Solution

To solve the system of equations and find the solution as an ordered pair, we’ll use the method of elimination. The given system of equations is: \[ \begin{cases} -5x - 5y = 25 & \\ 5x - 5y = -25 & \end{cases} \] Add the equations together to eliminate \(x\): \[ (-5x - 5y) + (5x - 5y) = 25 + (-25) \] Simplifying, we get: \[ 0 - 10y = 0 \] So, \[ -10y = 0 \] Divide both sides by \(-10\): \[ y = 0 \] Now substitute \(y = 0\) into one of the original equations to find \(x\). We’ll use the first equation: \[ -5x - 5(0) = 25 \] Simplifying, we have: \[ -5x = 25 \] Divide both sides by \(-5\): \[ x = -5 \] The solution to the system of equations is \((-5, 0)\).