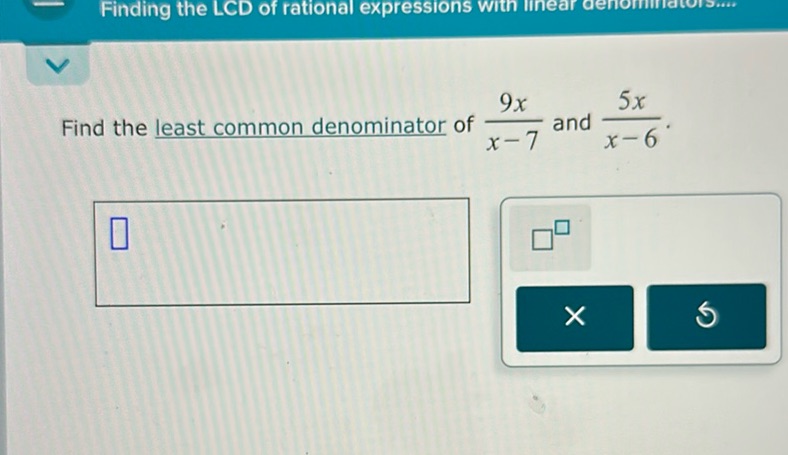
Question: Finding the LCD of rational expressions with linear denominators… Find the least common…

Finding the LCD of rational expressions with linear denominators… Find the least common denominator of $\frac{9x}{x-7}$ and $\frac{5x}{x-6}$.

Solution

To find the least common denominator (LCD) of the rational expressions \(\frac{9x}{x-7}\) and \(\frac{5x}{x-6}\), we need to determine the least common multiple (LCM) of their denominators. Step 1: Identify the denominators of each rational expression. The denominators are \(x - 7\) and \(x - 6\). Step 2: Find the LCM of the denominators. Since \(x - 7\) and \(x - 6\) are different linear expressions, the LCM is simply their product. \[ (x - 7)(x - 6) \] Therefore, the least common denominator is \((x - 7)(x - 6)\).