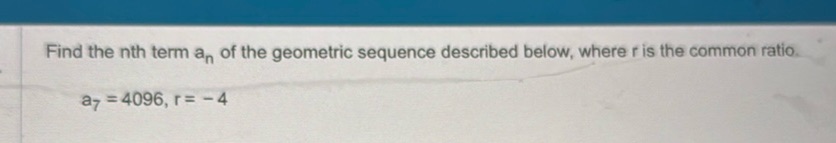
Question: Find the nth term \(a_n\) of the geometric sequence described below, where \(r\) is the common…

Find the nth term \(a_n\) of the geometric sequence described below, where \(r\) is the common ratio.

\(a_7 = 4096\), \(r = -4\)

Solution

To find the nth term \( a_n \) of a geometric sequence, we use the formula: \[ a_n = a_1 \cdot r^{n-1} \] Given: \( a_7 = 4096 \) and \( r = -4 \). First, we can write the expression for \( a_7 \): \[ a_7 = a_1 \cdot r^{6} \] Substitute the known values: \[ 4096 = a_1 \cdot (-4)^6 \] Calculate \( (-4)^6 \): \[ (-4)^6 = 4096 \] Substitute back into the equation: \[ 4096 = a_1 \cdot 4096 \] Solve for \( a_1 \): \[ a_1 = \frac{4096}{4096} = 1 \] Now, find the general formula for the nth term: \[ a_n = a_1 \cdot r^{n-1} = 1 \cdot (-4)^{n-1} \] Thus, the nth term is: \[ a_n = (-4)^{n-1} \]