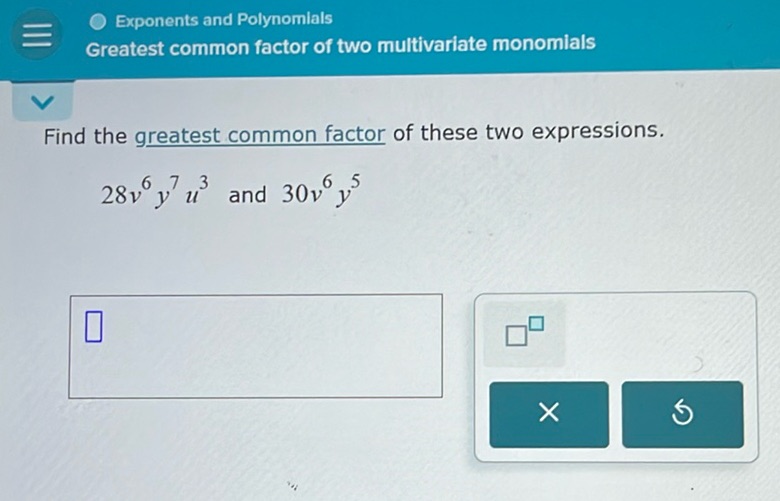
Question: Find the greatest common factor of these two expressions. \[ 28v^6y^7u^3 \quad \text{and}…

Find the greatest common factor of these two expressions.

\[ 28v^6y^7u^3 \quad \text{and} \quad 30v^6y^5 \]

Solution

To find the greatest common factor (GCF) of the expressions \(28v^6y^7u^3\) and \(30v^6y^5\), follow these steps: First, find the GCF of the coefficients: The coefficients are 28 and 30. The factors of 28 are 1, 2, 4, 7, 14, 28. The factors of 30 are 1, 2, 3, 5, 6, 10, 15, 30. The greatest common factor of 28 and 30 is 2. Next, find the GCF of the variable parts: For \(v\), compare the exponents: \[ v^6 \text{ and } v^6 \to \text{GCF is } v^6 \] For \(y\), compare the exponents: \[ y^7 \text{ and } y^5 \to \text{GCF is } y^5 \] For \(u\), since \(u\) is only present in the first term, it does not contribute to the GCF: \[ u^3 \to \text{GCF is } 1 \] Combine the GCFs of the coefficients and the variable parts: \[ 2v^6y^5 \] The greatest common factor of \(28v^6y^7u^3\) and \(30v^6y^5\) is \(2v^6y^5\).