Question: Fill in the blank to correctly complete the sentence. The solution set of the following system is…

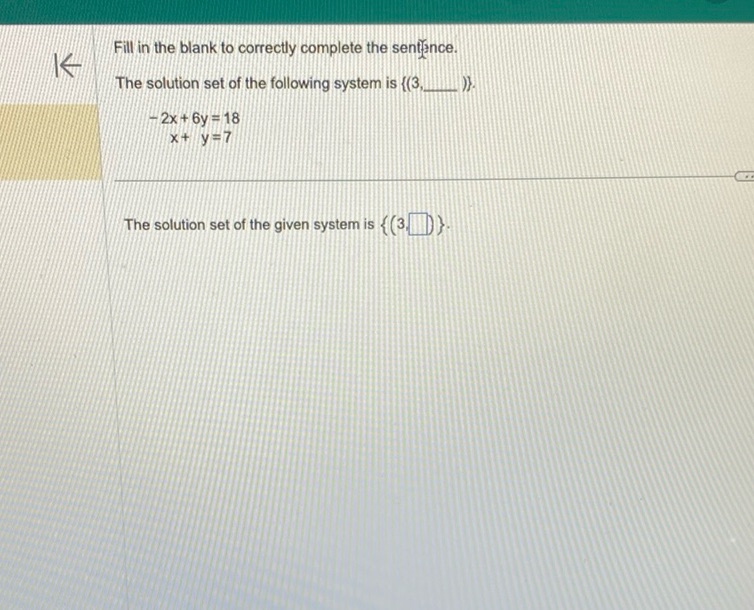
Fill in the blank to correctly complete the sentence.

The solution set of the following system is \{(3, \\\_)\).

\[ \begin{align*} -2x + 6y &= 18 \\ x + y &= 7 \end{align*} \]

The solution set of the given system is \(\{(3, \\\_)\}\).

Solution

To find the solution, we need to solve the system of equations: \[ \begin{cases} -2x + 6y = 18 & \\ x + y = 7 & \end{cases} \] First, solve the second equation for \(y\): \[ y = 7 - x \] Substitute \(y = 7 - x\) into the first equation: \[ -2x + 6(7-x) = 18 \] Simplify and solve for \(x\): \[ -2x + 42 - 6x = 18 \] Combine like terms: \[ -8x + 42 = 18 \] Subtract 42 from both sides: \[ -8x = 18 - 42 \] \[ -8x = -24 \] Divide by \(-8\): \[ x = 3 \] Now that we have \(x = 3\), substitute back to find \(y\): \[ y = 7 - x \] \[ y = 7 - 3 \] \[ y = 4 \] Thus, the solution set is \((3, 4)\). Fill in the blank with \(4\). The solution set of the given system is \(\{(3, 4)\}\).