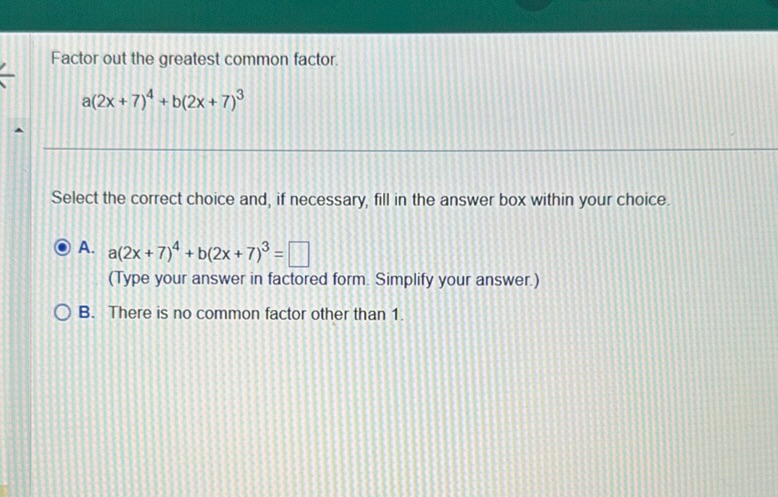
Question: Factor out the greatest common factor. a(2x + 7)^4 + b(2x + 7)^3 Select the correct choice and,…

Factor out the greatest common factor.

a(2x + 7)^4 + b(2x + 7)^3

Select the correct choice and, if necessary, fill in the answer box within your choice.

A. a(2x + 7)^4 + b(2x + 7)^3 = \boxed{\quad}

(Type your answer in factored form. Simplify your answer.)

B. There is no common factor other than 1.

Solution

To factor out the greatest common factor from \( a(2x + 7)^4 + b(2x + 7)^3 \), follow these steps: Identify the common factor in both terms, which is \( (2x + 7)^3 \). Factor out \( (2x + 7)^3 \) from each term: \[ a(2x + 7)^4 + b(2x + 7)^3 = (2x + 7)^3 [a(2x + 7) + b] \] Simplify the expression inside the brackets: \[ = (2x + 7)^3 [a(2x + 7) + b] = (2x + 7)^3 [2ax + 7a + b] \] The expression in factored form is: \[ = (2x + 7)^3 (2ax + 7a + b) \]