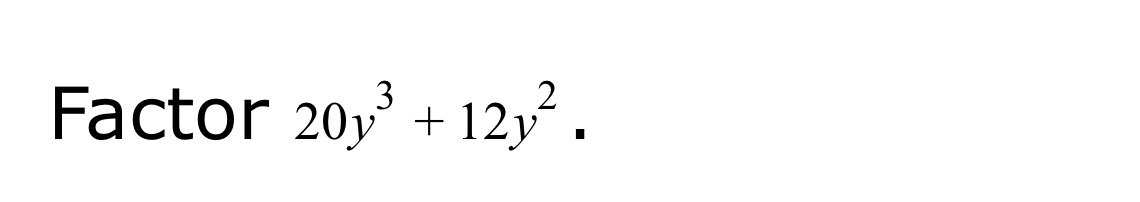
Question: Factor $20y^3 + 12y^2$.

Solution

The problem is to factor the expression \(20y^3 + 12y^2\). First, identify the greatest common factor (GCF) of the terms. The GCF of the coefficients 20 and 12 is 4. Next, the smallest power of \(y\) that appears in both terms is \(y^2\). Therefore, the GCF is \(4y^2\). Factor out \(4y^2\) from the expression: \[ 20y^3 + 12y^2 = 4y^2(5y + 3) \] The expression is factored as \(4y^2(5y + 3)\).