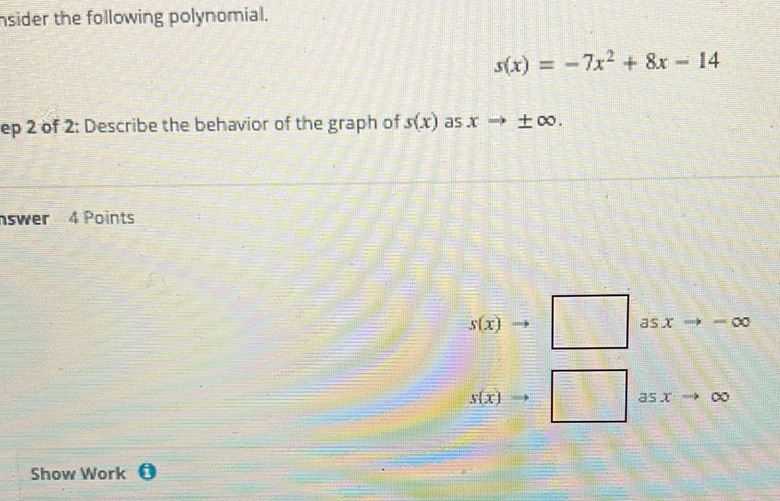
Question: Consider the following polynomial. \[ s(x) = -7x^2 + 8x - 14 \] Step 2 of 2: Describe the…

Consider the following polynomial.

\[ s(x) = -7x^2 + 8x - 14 \]

Step 2 of 2: Describe the behavior of the graph of \( s(x) \) as \( x \to \pm \infty \).

Answer 4 Points

\[ s(x) \to \boxed{\phantom{as x \to -\infty}} \quad \text{as} \quad x \to -\infty \]

\[ s(x) \to \boxed{\phantom{as x \to \infty}} \quad \text{as} \quad x \to \infty \]

Show Work

Solution

Identify the leading term of the polynomial. \[ s(x) = -7x^2 + 8x - 14 \] The leading term is \(-7x^2\). Determine the end behavior based on the leading term. Since the coefficient of \(x^2\) is negative, the parabola opens downward. As \(x \to -\infty\), \(s(x) \to -\infty\). As \(x \to \infty\), \(s(x) \to -\infty\).