Question: Consider the following polynomial function. \[ f(x) = x^4 + x^3 - 11x^2 - 5x + 30 \] Step 3 of…

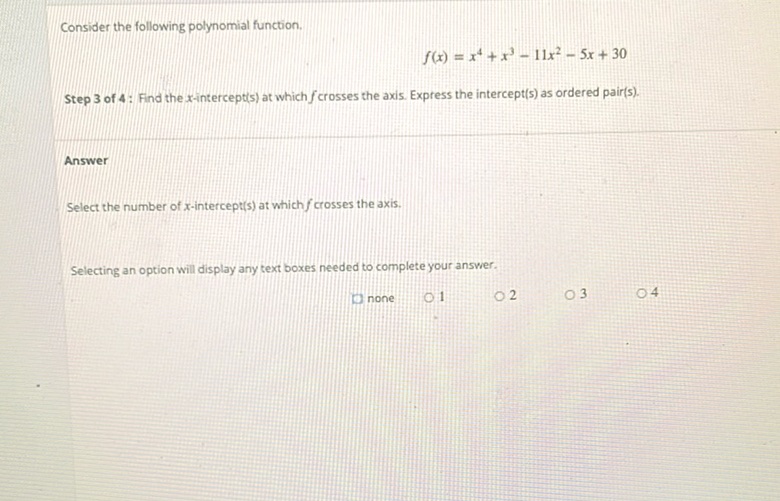
Consider the following polynomial function.

\[ f(x) = x^4 + x^3 - 11x^2 - 5x + 30 \]

Step 3 of 4: Find the x-intercept(s) at which \( f \) crosses the axis. Express the intercept(s) as ordered pair(s).

Answer

Select the number of x-intercept(s) at which \( f \) crosses the axis.

Selecting an option will display any text boxes needed to complete your answer.

• none
• 1
• 2
• 3
• 4

Solution

First, simplify the polynomial function. \[ f(x) = x^3 + x^2 - 11x^2 - 5x + 30 \] \[ f(x) = x^3 - 10x^2 - 5x + 30 \] Next, find the x-intercepts by setting \( f(x) = 0 \). \[ x^3 - 10x^2 - 5x + 30 = 0 \] Attempt to find rational roots using the Rational Root Theorem. The possible rational roots are \( \pm1, \pm2, \pm3, \pm5, \pm6, \pm10, \pm15, \pm30 \). Testing these values, none satisfy the equation. Since no rational roots are found, use calculus to determine the number of real roots. Find the derivative of \( f(x) \): \[ f’(x) = 3x^2 - 20x - 5 \] Set the derivative equal to zero to find critical points. \[ 3x^2 - 20x - 5 = 0 \] Solve for \( x \): \[ x = \frac{20 \pm \sqrt{(20)^2 - 4 \cdot 3 \cdot (-5)}}{2 \cdot 3} \] \[ x = \frac{20 \pm \sqrt{400 + 60}}{6} \] \[ x = \frac{20 \pm \sqrt{460}}{6} \] \[ x \approx \frac{20 \pm 21.45}{6} \] This gives two critical points at approximately \( x \approx 6.91 \) and \( x \approx -0.24 \). Evaluate \( f(x) \) at these points: For \( x \approx -0.24 \): \[ f(-0.24) \approx 31.14 \] For \( x \approx 6.91 \): \[ f(6.91) \approx -226.54 \] Since \( f(x) \) changes from positive to negative, there is only one real root. Final Answer: The polynomial function crosses the x-axis at one x-intercept.