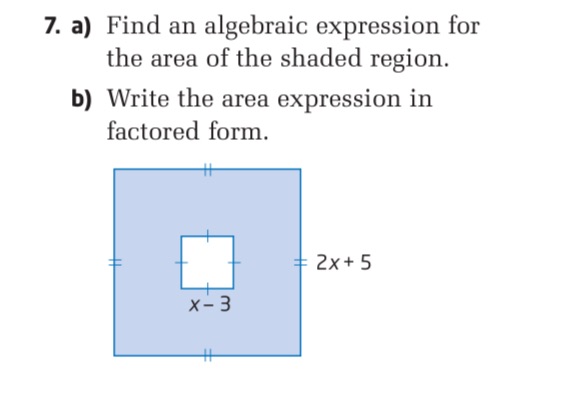
Question: 7. a) Find an algebraic expression for the area of the shaded region. b) Write the area expression…

7. a) Find an algebraic expression for the area of the shaded region.

b) Write the area expression in factored form.

[Image description: A large square with side length \(2x + 5\) and a smaller square inside it with side length \(x - 3\). The shaded region is the area outside the smaller square but inside the larger square.]

Solution

a) Find an algebraic expression for the area of the shaded region. Step 1: Find the area of the outer square. \[ A_{outer} = (2x + 5)^2 & \] Step 2: Find the area of the inner square. \[ A_{inner} = (x - 3)^2 & \] Step 3: Subtract the inner area from the outer area. \[ A_{shaded} = (2x + 5)^2 - (x - 3)^2 & \] b) Write the area expression in factored form. Step 1: Expand the squared terms. \[ (2x + 5)^2 = 4x^2 + 20x + 25 & \] \[ (x - 3)^2 = x^2 - 6x + 9 & \] Step 2: Subtract the inner area from the outer area. \[ A_{shaded} = 4x^2 + 20x + 25 - (x^2 - 6x + 9) = 3x^2 + 26x + 16 & \] Step 3: Factor the quadratic expression. \[ 3x^2 + 26x + 16 = (3x + 2)(x + 8) & \]