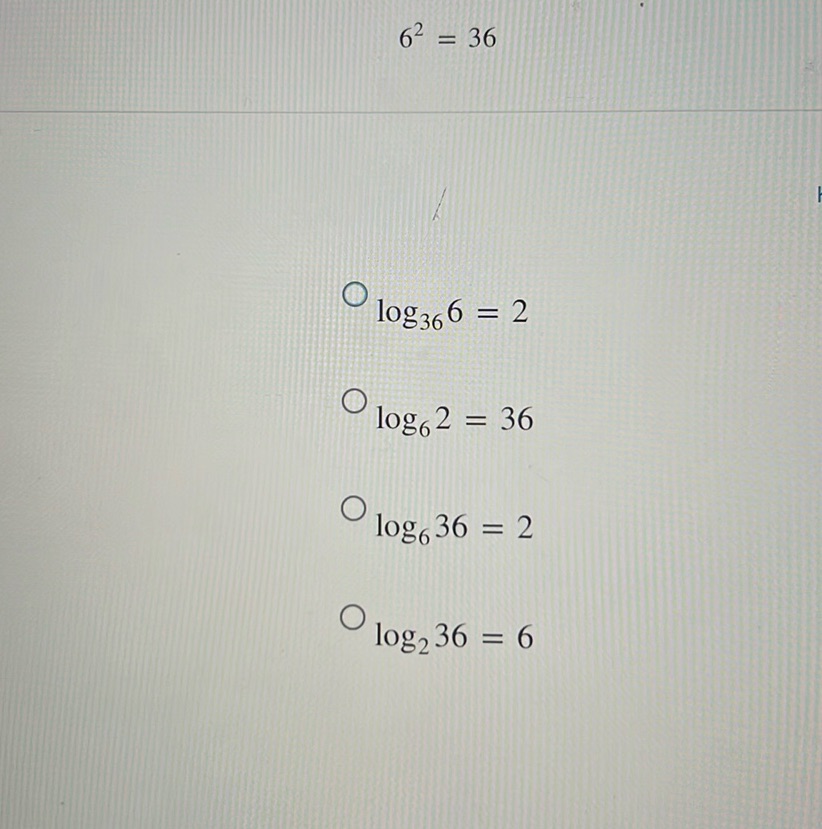
Question: 6^2 = 36 - \(\log_{36} 6 = 2\) - \(\log_{6} 2 = 36\) - \(\log_{6} 36 = 2\) - \(\log_{2}…

6^2 = 36

• \(\log_{36} 6 = 2\)
• \(\log_{6} 2 = 36\)
• \(\log_{6} 36 = 2\)
• \(\log_{2} 36 = 6\)

Solution

To solve the problem, we need to express the equation \(6^2 = 36\) in logarithmic form. Here, 6 is the base, and 36 is the result. The logarithm describes the power to which the base must be raised to obtain the result. Convert \(6^2 = 36\) to its logarithmic form: \[ \log_6 36 = 2 \] Thus, the correct option is: \[ \log_6 36 = 2 \]