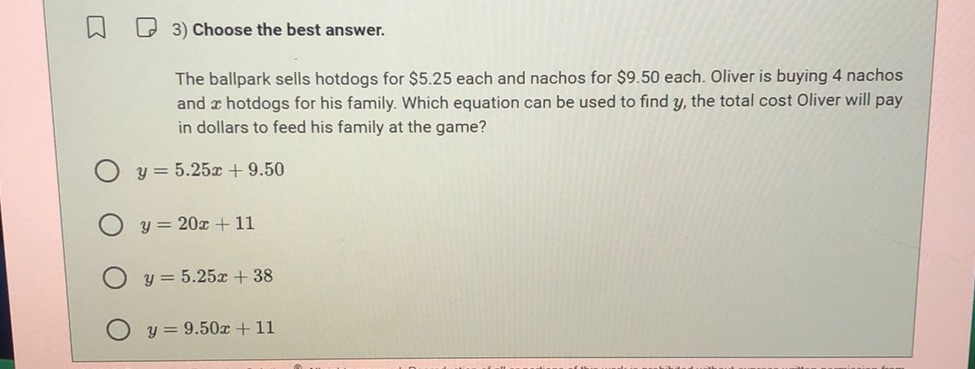
Question: 3) Choose the best answer. The ballpark sells hotdogs for $5.25 each and nachos for $9.50 each…

3. Choose the best answer.

The ballpark sells hotdogs for $5.25 each and nachos for $9.50 each. Oliver is buying 4 nachos and \( x \) hotdogs for his family. Which equation can be used to find \( y \), the total cost Oliver will pay in dollars to feed his family at the game?

• \( y = 5.25x + 9.50 \)
• \( y = 20x + 11 \)
• \( y = 5.25x + 38 \)
• \( y = 9.50x + 11 \)

Solution

The problem states that hotdogs cost $5.25 each and nachos cost $9.50 each. Oliver is buying 4 nachos and \( x \) hotdogs. We need to find the total cost \( y \). First, calculate the cost for 4 nachos: \[ \text{Cost of nachos} = 4 \times 9.50 \] Calculate the cost for \( x \) hotdogs: \[ \text{Cost of hotdogs} = 5.25x \] Add the two costs to find the total cost \( y \): \[ y = 5.25x + 4 \times 9.50 \] \[ y = 5.25x + 38 \] The equation that represents the total cost is: \[ y = 5.25x + 38 \] The correct answer is the option \( y = 5.25x + 38 \).