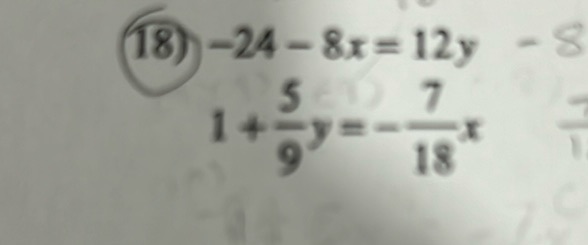
Question: (18) \(-24 - 8x = 12y\) \(1 + \frac{5}{9}y = -\frac{7}{18}x\)

(18) \(-24 - 8x = 12y\)

\(1 + \frac{5}{9}y = -\frac{7}{18}x\)

Solution

To solve the system of equations, let’s write them down first: \[ \begin{cases} -24 - 8x = 12y & \\ 1 + \frac{5}{9}y = -\frac{7}{18}x & \end{cases} \] Step 1: Simplify the first equation Add 24 to both sides: \[ -8x = 12y + 24 \] Divide the entire equation by 4 to simplify: \[ -2x = 3y + 6 \] Step 2: Simplify the second equation Subtract 1 from both sides: \[ \frac{5}{9}y = -\frac{7}{18}x - 1 \] Clear the fractions by multiplying the entire equation by 18: \[ 10y = -7x - 18 \] Step 3: Solve the system using substitution or elimination Rearrange the first equation \(-2x = 3y + 6\) for \(x\): \[ x = -\frac{3}{2}y - 3 \] Substitute \(x\) in the second equation: \[ 10y = -7\left(-\frac{3}{2}y - 3\right) - 18 \] Distribute \(-7\): \[ 10y = \frac{21}{2}y + 21 - 18 \] Convert to a common base and simplify: \[ 10y = \frac{21}{2}y + 3 \] Multiply everything by 2 to clear the fraction: \[ 20y = 21y + 6 \] Move \(21y\) to the other side: \[ -1y = 6 \] Solve for \(y\): \[ y = -6 \] Step 4: Solve for \(x\) Substitute \(y = -6\) back into \(x = -\frac{3}{2}y - 3\): \[ x = -\frac{3}{2}(-6) - 3 \] Calculate: \[ x = 9 - 3 \] \[ x = 6 \] The solution to the system is \(x = 6\) and \(y = -6\).